Advertisements
Advertisements
Question
The following table shows points on a number line and their co-ordinates. Decide whether the pair of segments given below the table are congruent or not.
| Point | A | B | C | D | E |
| Co-ordinate | -3 | 5 | 2 | -7 | 9 |
seg BE and seg AD
Solution
The co-ordinates of points B and E are 5 and 9 respectively.
We know that 9 > 5
∴ l(BE) = 9 − 5
∴ l(BE) = 4
The co-ordinates of points A and D are −3 and −7 respectively.
We know that − 3 > − 7
∴ l(AD) = − 3 − (− 7)
∴ l(AD) = − 3 + 7
∴ l(AD) = 4
∴ l(BE) = l(AD)
∴ seg BE and seg AD are congruent.
APPEARS IN
RELATED QUESTIONS
Point P is the midpoint of seg CD. If CP = 2.5, find l(CD).
How many line segments are there in the given line? Name them.![]()
If line PQ || line m, then line segment PQ || m.
Name all the line segments in figure
Is it possible for the same line segment to have two different lengths?
In which of the following figures, perpendicular bisector is shown?
How many line segments are there in given figure?
In given figure how many line segments are there? Name them.
Consider the following figure of line \[\overleftrightarrow{MN}\]. Say whether the following statement is true or false in the context of the given figure.
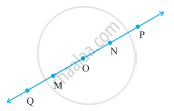
M, O, and N are points on a line segment `overline("MN")`.
Consider the following figure of line \[\overleftrightarrow{MN}\]. Say whether the following statement is true or false in the context of the given figure.

M and N are the end points of line segment `overline"MN"`.
