Question

The function y = x²e^–x is decreasing in the interval

Options

• (0, 2)

• (2, ∞)

• (–∞, 0)

• (–∞, 0) ∪ (2, ∞)

Answer 1

(–∞, 0) ∪ (2, ∞)

Explanation:

We have,

f(x) = y = x²e^–x 

∴ `dy/dx = 2x e^-x + x^2(-1)e^-x = xe^-x(2 - x)`

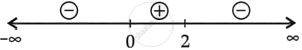
Now, put `dy/dx = 0`

`\implies` x = 0 and x = 2

The points x = 0 and x = 2 divide the real line into three disjoint intervals i.e., (–∞, 0), (0, 2) and (2, ∞)

In intervals, (–∞, 0) and (2, ∞), f'(x) < 0 as e^–x is always positive.

∴ f(x) or y is decreasing in (–∞, 0) and (2, ∞).