Question

The given figure shows two circles with centres A and B; and radii 5 cm and 3 cm respectively, touching each other internally. If the perpendicular bisector of AB meets the bigger circle in P and Q, find the length of PQ.

Answer 1

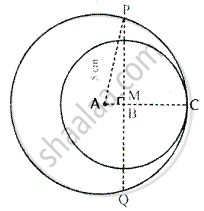
Join AP and produce AB to meet the bigger circle at C.
AB = AC – BC = 5 cm – 3 cm = 2 cm But, M is the mid – point of AB.
∴ `AM = 2/2 = 1cm`
Now in right ∆APM,

`AP^2 = MP^2 + AM^2`     [Pythagoras Theorem]
⇒ ` (5)^2 = MP^2 +1`
⇒ `25 = MP^2 1`
⇒ ` MP^2 = 25 -1`

⇒ `MP^2 = 24`

⇒ ` MP =sqrt24 =sqrt(4 xx 6 )= 2sqrt 6 cm`

∴ `PQ = 2MP = 2xx 2sqrt 6 = 4sqrt 6 cm`
    ⇒ ` PQ = 4 xx 2.45 = 9.8 cm`