Advertisements
Advertisements
Question
The maximum of z = 5x + 2y, subject to the constraints x + y ≤ 7, x + 2y ≤ 10, x, y ≥ 0 is ______.
Options
10
26
35
70
MCQ
Fill in the Blanks
Solution
The maximum of z = 5x + 2y, subject to the constraints x + y ≤ 7, x + 2y ≤ 10, x, y ≥ 0 is 35.
Explanation:
Feasible region lies on origin side of x + y = 7 and x + 2y = 10, and in first quadrant.
The corner points of feasible region are 0(0, 0), A(7, 0), E(4, 3) and D(O, 5)
∴ Maximum z = 5x + 2y is at A(7, 0)
∴ Maximum, z = 5(7) + 2(0) = 35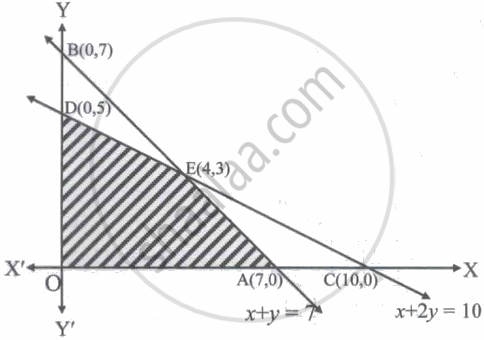
shaalaa.com
Is there an error in this question or solution?
