Question

The maximum value of Z = 5x + 4y, Subject to y ≤ 2x, x ≤ 2y, x + y ≤ 3, x ≥ 0, y ≥ 0 is ______.

Options

• 14

• 12

• 13

• 18

Answer 1

The maximum value of Z = 5x + 4y, Subject to y ≤ 2x, x ≤ 2y, x + y ≤ 3, x ≥ 0, y ≥ 0 is 14.

Explanation:

We have, z = Sx + 4y

Subject to contraints y ≤ 2x, x ≤ 2y,

x + y ≤ 3, x ≥ 0, y ≥ 0

On taking given constraints as equations, we get the following graph.

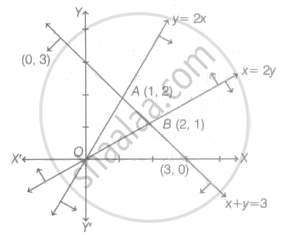
Intersecting point of line y = 2x and x + y = 3 is A(1, 2) and intersecting point of line x = 2y and x + y = 3 is B (2, 1).

Here, OABO is the required feasible region

whose corner points are 0(0, 0), A(1, 2) and B(2, 1).

Corner points	Z = 5X + 4y
O(0, 0)	5 × 0 + 4 × 0 = 0
A(1, 2)	5 × 1 + 4 × 2 = 13
B(2, 1)	5 × 2 + 4 × 1 = 14 (maxmium)