Advertisements
Advertisements
Question
The minimum value of z = 7x + 9y subject to 3x + y ≤ 6, 5x + 8y ≤ 40, x ≥ 0, y ≥ 2 is ______.
Options
45
18
9
`82/3`
MCQ
Fill in the Blanks
Solution
The minimum value of z = 7x + 9y subject to 3x + y ≤ 6, 5x + 8y ≤ 40, x ≥ 0, y ≥ 2 is 18.
Explanation:
Feasible region lies on origin side of lines 3x + y = 6 and 5x + 8y = 40 and above line y = 2, in first quadrant.
The corner points of the feasible region
`"A"(0, 2), "B"(4/3, 2), "C"(8/19, 90/19)` and D(0, 5)
At A(0, 2), z = 18
At `"B"(4/3, 2)`, z = `82/3`
At c = `(8/19, 9/19)`, z = `866/19`
At D(0, 5), z = 45
∴ Minimum value of z is 18.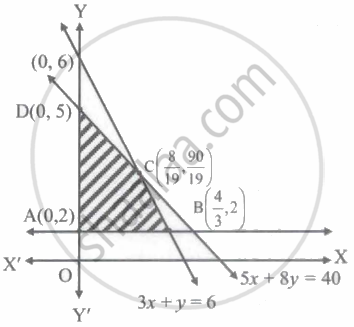
shaalaa.com
Is there an error in this question or solution?
