Question

The moment of inertia of a uniform square plate about an axis perpendicular to its plane and passing through the centre is `"Ma"^2/6` where M is the mass and 'a' is the side of square plate. Moment of inertia of this plate about an axis perpendicular to its plane and passing through one of its corner is ______.

Options

• `(2"Ma"^2)/3`

• `("Ma"^2)/3`

• `3/("Ma"^2)`

• `(3"Ma"^2)/2`

Answer 1

The moment of inertia of a uniform square plate about an axis perpendicular to its plane and passing through the centre is `"Ma"^2/6` where M is the mass and 'a' is the side of square plate. Moment of inertia of this plate about an axis perpendicular to its plane and passing through one of its corner is `underline((2"Ma"^2)/3)`.

Explanation:

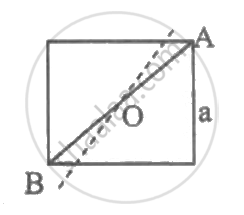
`I_circ = "Ma"^2/6`

`"AB"=sqrt(2a^2)=sqrt2a`

`"AO"=a/sqrt2=h`

`therefore I_A=I_circ+"Mh"^2`

`="Ma"^2/6+"Ma"^2/2=("Ma"^2+3"Ma"^2)/6=(4"Ma"^2)/6=(2"Ma"^2)/3`