Question

The quadrilateral formed by joining the angle bisectors of a cyclic quadrilateral is a ______ 

Options

• cyclic quadrilateral

• parallelogram

• square

• Rectangle

Answer 1

The quadrilateral formed by joining the angle bisectors of a cyclic quadrilateral is a cyclic quadrilateral.

Explanation:

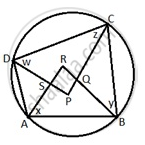
ABCD is a cyclic quadrilateral.

∴ ∠A + ∠C = 180° and ∠B + ∠D = 180°

`1/2`∠A + `1/2`∠C = 90° and `1/2`∠B + `1/2`∠D = 90°

x + z = 90° and y + w = 90°

In ΔARB and ΔCPD,

x + y + ∠ARB = 180° and z + w + ∠CPD = 180°

∠ARB = 180° - (x + y) and ∠CPD = 180° - (z + w)

∠ARB + ∠CPD = 360° - (x + y + z + w) = 360° - (90 + 90)

= 360° - 180° = 180°

∠ARB + ∠CPD = 180°

∠SRQ + ∠QPS = 180°

The sum of a pair of opposite angles of a quadrilateral PQRS is 180°.

Hence PQRS is a cyclic quadrilateral.