Advertisements
Advertisements
Question
The ratio of volumes of two cones is 4 : 5 and the ratio of the radii of their bases is 2:3. Find the ratio of their vertical heights.
Solution
Let ratio of radius be 'r '
Radius of `1^(st)`cone = 2r
Radius of `2^(nd)`cone = 3r
Similarly
Let volume ratio be ‘v’
Volume of `1^(st)` cone→ 4v
Similarly volume of `2^(nd)` cone → 5v
∴`V_1/V_2=(4v)/(5v)=4/5`
⇒ `(1/3pir_1^2h_1)/(1/3pir_1^2)=4/5`
⇒`( h_1(2r)^2)/(h_2(3r)^2)=4/5`
⇒` h_1/h_2xx(4r^2)/(9r^2)=4/5`
⇒ `h_1/h_2xx36/20=18/20=9/5`
∴ Ratio of the inner height is 9:5
APPEARS IN
RELATED QUESTIONS
The following figure represents a solid consisting of a right circular cylinder with a hemisphere at one end and a cone at the other. This common radius is 7 cm. The height of the cylinder and cone are each of 4 cm. Find the volume of the solid.

The area of the curved surface of a cone is 60 cm2. If the slant height of the cone be 8 cm, find the radius of the base?
A right angled triangle of which the sides containing he right angle are 6.3 cm and lo cm in length, is made to turn round on the longer side. Find the volume of the solid, thus generated. Also, find its curved surface area.
The volume of a right circular cone is 9856 cm3. If the diameter of the base is 28 cm, find:
(i) height of the cone (ii) slant height of the cone (iii) curved surface area of the cone.
A solid metal sphere is cut through its center into 2 equal parts. If the diameter of the sphere is `3 1/2 cm`, find the total surface area of each part correct to two decimal places.
A solid metallic hemisphere of diameter 28 cm is melted and recast into a number of identical solid cones, each of diameter 14 cm and height 8 cm. Find the number of cones so formed.
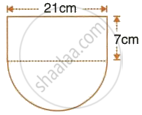
The given figure shows the cross section of a water channel consisting of a rectangle and a semi-circle. Assuming that the channel is always full, find the volume of water discharged through it in one minute if water is flowing at the rate of 20 cm per second. Give your answer in cubic metres correct to one place of decimal.
Find the height of the cone whose base radius is 5 cm and volume is 75π cm3.
The total surface area of a right circular cone of slant height 13 cm is 90π cm2. Calculate: its volume in cm3. Take π = 3.14
The surface area of a solid sphere is increased by 21% without changing its shape. Find the percentage increase in its: volume
