Question

The rod given in the figure is made up of two different materials.

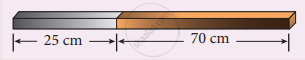
Both have square cross-sections of 3 mm side. The resistivity of the first material is 4 x 10^-3 Ω.m and it is 25 cm long while the second material has a resistivity of 5 x 10^-3 Ω.m and is of 70 cm long. What is the resistivity of rod between its ends?

Answer 1

Square cross section of side, a = 3 mm = 3 x 10^-3 m

Cross section of side, A = a² = 9 x 10⁶ m

First material:

Resistivity of the material, ρ₁ = 4 x 10^-3 Ωm

length, l₁ = 25 cm = 25 x 10^-2 m

Resistance of the lord, R₁ = `(rho_1l_1)/"A"`

`= (4xx10^-3 xx 25 xx 10^-2)/(9 xx 10^-6)`

`= (100xx10^-5xx10^6)/9`

R₁ = 11.11 x 10¹ Ω

Second material:

Resistivity of the material, ρ₂ = 5 x 10^-3 Ωm

length, l₂ = 70 cm = 70 x 10^-2 m

Resistance of the rod, R₂ = `(rho_2l_2)/"A"`

`= (5 xx 10^-3 xx 70 xx 10^-2)/(9 xx 10^-6)`

`= (350 xx 10^-5 xx 10^6)/9`

R₂ = 38.88 x 10¹

R₂ = 389 Ω

Total resistance between the ends f the rods

R = R₁ + R₂ = 111 + 389

= 500 Ω