Advertisements
Advertisements
Question
Two circles of radii 5.5 cm and 4.2 cm touch each other externally. Find the distance between their centres.
Solution
Let the two circles having centres P and R touch each other externally at point Q.
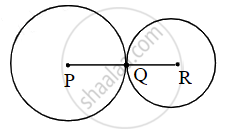
Here, PQ = 5.5 cm, QR = 4.2 cm
The two circles touch each other externally. ...(P−Q−R)
If the circles touch each other externally, distance between their centres is equal to the sum of their radii.
∴ By theorem of touching circles,
∴ PR = PQ + QR
∴ PR = 5.5 + 4.2
∴ PR = 9.7 cm
∴ The distance between the centres of the circles is 9.7 cm.
APPEARS IN
RELATED QUESTIONS
If two circles with radii 5 cm and 3 cm respectively touch internally, find the distance between their centres.
If two circles with radii 8 cm and 3 cm, respectively, touch internally, then find the distance between their centers.
If radii of two circles are 4 cm and 2.8 cm. Draw a figure of this circles touching each other externally.
In the given figure, the circles with centres P and Q touch each other at R. A line passing through R meets the circles at A and B respectively. Prove that – (1) seg AP || seg BQ,
(2) ∆APR ~ ∆RQB, and
(3) Find ∠ RQB if ∠ PAR = 35°
In the given figure, ray PQ touches the circle at point Q. PQ = 12, PR = 8, find PS and RS.
Four alternative answers for the following question is given. Choose the correct alternative.
Two circles of radii 5.5 cm and 3.3 cm respectively touch each other. What is the distance between their centers ?
Four alternative answers for the following question is given. Choose the correct alternative.
Two circles intersect each other such that each circle passes through the centre of the other. If the distance between their centres is 12, what is the radius of each circle?
Four alternative answers for the following question is given. Choose the correct alternative.
Chords AB and CD of a circle intersect inside the circle at point E. If AE = 5.6, EB = 10, CE = 8, find ED.
Line l touches a circle with centre O at point P. If radius of the circle is 9 cm, answer the following.
- What is d(O, P) = ? Why?
- If d(O, Q) = 8 cm, where does the point Q lie?
- If d(O, Q) = 15 cm, How many locations of point Q are line on line l? At what distance will each of them be from point P?

How many common tangents can be drawn to two circles which touch each other internally?
(A) One (B) Two (C) Three (D) Four
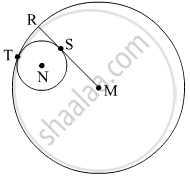
In the given figure, circle with centre M touches the circle with centre N at point T. Radius RM touches the smaller circle at S. Radii of circles are 9 cm and 2.5 cm. Find the answers to the following questions hence find the ratio MS:SR.
(1) Find the length of segment MT
(2) Find the length of seg MN
(3) Find the measure of ∠NSM.
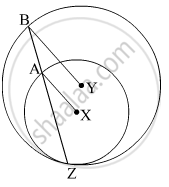
In the given figure, circles with centres X and Y touch internally at point Z . Seg BZ is a chord of bigger circle and it itersects smaller circle at point A. Prove that, seg AX || seg BY.
If two circles with diameters 8 cm and 6 cm respectively touch externally, find the distance between their centers.
Four alternative answers for the following question is given. Choose the correct alternative.
Two circles having diameters 8 cm and 6 cm touch each other internally. Find the distance between their centres.
Line ℓ touches a circle with centre O at point P. If radius of the circle is 9 cm, answer the following.
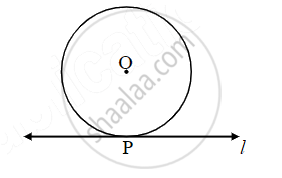
What is d(O, P) =? Why?
Line ℓ touches a circle with centre O at point P. If radius of the circle is 9 cm, answer the following.

If d(O, R) = 15 cm, how many locations of point R are line on line l? At what distance will each of them be from point P?
A circle with centre P is inscribed in the ∆ABC. Side AB, side BC, and side AC touch the circle at points L, M, and N respectively. The radius of the circle is r.
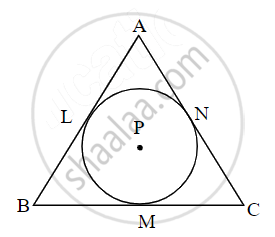
Prove that: A(ΔABC) = `1/2` (AB + BC + AC) × r
