Advertisements
Advertisements
Question
Two wheels of the moment of inertia 4 kgm2 rotate side by side at the rate of 120 rev/min and 240 rev/min respectively in the opposite directions. If now both the wheels are coupled by means of a weightless shaft so that both the wheels rotate with a common angular speed. Calculate the new speed of rotation.
Solution
I1 = I2 = I = 4 kg m2 , n1 = 120 r.p.m, n2 = 240 r.p.m.
Initially, the angular velocities of the two wheels are `vecω_1` and `vecω_2` and, therefore the angular momentum `vecL_1` and `vecL_2` are in opposite directions.
∴ The magnitude of the total initial angular momentum, L = -L1 + L2
∴ L = -Iω1 + Iω2 .................(i)
After coupling on the same shaft, the total moment of inertia is 2I.
Let, ω = 2πn be the common angular speed.
∴ The magnitude of the total final angular momentum L′ = 2Iω ….(ii)
By the principle of conservation of angular momentum, L = L′
∴ Equating equation (i) and (ii), we have
I(ω2 − ω1) = 2Iω
∴ 2 × 2πn = 2π(n2 - n1)
∴ 2n = n2 − n1
∴ n = `(n_2 - n_1)/2 = (240 - 120)/2` = 60 r.p.m.
The new speed of rotation of the wheels would be 60 r.p.m.
APPEARS IN
RELATED QUESTIONS
The moment of inertia of a circular loop of radius R, at a distance of R/2 around a rotating axis parallel to horizontal diameter of the loop is ______
The moment of inertia of a uniform circular disc about a tangent in its own plane is 5/4MR2 where M is the mass and R is the radius of the disc. Find its moment of inertia about an axis through its centre and perpendicular to its plane.
A particle starting from rest moves along the circumference of a circle of radius r with angular acceleration a. The magnitude of the average velocity, in the time it completes the small angular displacement θ is
Surface density of charge on a charged conducting sphere of radius R in terms of electric field intensity E at a distance r in free space is ____________.
(r > R, ε0 = permittivity of free space)
The moment of inertia of a uniform thin rod of length L and mass M about an axis passing through a point at a distance of L/3 from one of its ends and perpendicular to the rod is ______
If radius of the solid sphere is doubled by keeping its mass constant, the ratio of their moment of inertia about any of its diameter is ______.
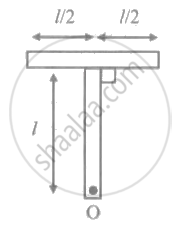
Two rods, each of mass m and length l, are joined as shown in the figure. Moment of inertia of system about an axis passing through one end of the rod, i.e. O, and perpendicular to the plane is ____________.
Four solid spheres each of mass M and radius R are placed with their centres on the four comers of a rectangle. If b is a breadth of rectangle and its length is twice of its breadth, then find moment of inertia of the system about an axis along one of the breadth of the rectangle.
Three identical rods each of mass 'M' and length 'L' are joined to form a symbol 'H'. The moment of inertia of the system about one of the sides of 'H' is ______.
A particle is performing U.C.M. along the circumference of a circle of diameter 50 cm with frequency 2 Hz. The acceleration of the particle in m/s2 is ______.
A flywheel of mass 20 kg and radius 5 cm is revolving at a speed of 300 rpm. Its kinetic energy is ______.
From a disc of mass 'M' and radius 'R', a circular hole of diameter 'R' is cut whose rim passes through the center. The moment of inertia of the remaining part of the ruse about perpendicular axis passing through the center is ______.
A cord is wound round the circumference of a wheel of radius 'r'. The axis of the wheel is horizontal and moment of inertia about it is T. A block of mass 'm' is attached to free end of the cord, initially at rest. When the wheel rotates and the block moves vertically downwards through distance 'h', the angular velocity of the wheel will be ______.
(Neglect the mass of cord, g =acceleration due to gravity)
The moment of inertia of a body initially at rest about a given axis is 1.2 kg m2. On applying an acceleration of 25 rad/s2, the time it will take to acquire a rotational kinetic energy of 1500 J is ____________.
Two discs having moment of inertia I1 and I2 are made from same material have same mass. Their thickness and radii are t1, t2, and R1, R2 respectively. The relation between moment of inertia of each disc about an axis passing through its centre and perpendicular to its plane and its thickness is ______.
Figure shows triangular lamina which can rotate about different axis of rotation. Moment of inertia is maximum about the axis ______.
Moment of inertia of the rod about an axis passing through the centre and perpendicular to its length is 'I1'. The same rod is bent into a ring and its moment of inertia about the diameter is 'I2', then `"I"_2/"I"_1` is ______.
A uniform disc of mass 4 kg has radius of 0.4 m. Its moment of inertia about an axis passing through a point on its circumference and perpendicular to its plane is ______.
A thin circular ring of mas 'M' and radius 'R' is rotating about a transverse axis passing through its centre with constant angular velocity 'ω'. Two objects each of mass 'm' are attached gently to the opposite ends of a diameter of the ring. What is the new angular velocity?
For the same cross-sectional area and for a given load, the ratio of depressions for the beam of a square cross-section and circular cross-section is ______.
The moment of inertia of a body about a given axis is 1.2 kg-m2. Initially, the body is at rest. In order to produce, a rotational kinetic energy of 1500 J, an acceleration of 25 rad/s2 must be applied about that axis for a duration of ______.
The moment of inertia (MI) of a disc of radius R and mass M about its central axis is ______.
