Question

Use Gauss's law to obtain the expression for the electric field due to a uniformly charged infinite plane sheet of charge.

Answer 1

Let us consider an infinitely thin plane sheet of positive charge having a uniform surface charge density σ. Let P be the point where the electric field E is to be found. Let us imagine a cylindrical Gaussian surface of length 2r and containing P, as shown. The net flux is through the cylindrical Gaussian surface.

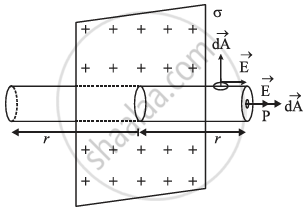
`phi = "∮"vecE*vec(dA)`

= `\underset(RCF)intvecE*vec(dA) + \underset(LCF)intvecE*vec(dA) + \underset(CS)intvecE*vec(dA)`

= `\underset(RCF)intE*dAcos0"°" + \underset(LCF)intE*dAcos0"°" + \underset(CS)intE*dAcos90"°"`

= EA + EA + 0

= 2EA   ...(1)

Here, A is the area of the cross-section of each circular face, i.e., LCF and RCF.

The total charge enclosed by the Gaussian cylinder

= σA   ...(2)

Using Gauss’s theorem, from (1) and (2),

2EA = `(sigmaA)/epsilon_0`

E = `sigma/(2epsilon_0`