Advertisements
Advertisements
Question
Verify Euler's formula for the following polyhedron:

Solution
In the following polyhedron:
Edges E=21
Faces F=9
Vertices V=14
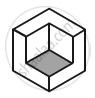
Now, putting these values in Euler's formula:
LHS: F+V
= 9+14
= 23
RHS: E+2
=21+2
=23
Thisis true.
Hence, Euler's formula is satisfied.
APPEARS IN
RELATED QUESTIONS
Can a polyhedron have for its faces 3 triangles?
Is it possible to have a polyhedron with any given number of faces? (Hint: Think of a pyramid).
Here is an incomplete net for making a cube. Complete it in at least two different ways. Remember that a cube has six faces. How many are there in the net here? (Give two separate diagrams. If you like, you may use a squared sheet for easy manipulation.)
What is the least number of planes that can enclose a solid? What is the name of the solid?
Is a square prism same as a cube?
Verify Euler’s formula for the table given below.
| Faces | Verticles | Edges |
| 12 | 20 | 30 |
How many faces does the following solid have?
Tetrahedron
How many edges does the following solid have?
Sphere
How many faces does figure have?

Find the number of faces in the given shape:

