Question

What is the area of the region bounded by the curve `y^2 = 4x` and the line `x` = 3.

Options

• `8sqrt(3)` units

• `sqrt(3)` units

• 8 units

• `- 8 sqrt(3)` units

Answer 1

`8sqrt(3)` units

Explanation:

As shown in the figure, the curve `y^2 = 4x` is a parabola.

The x-axis is the parabola's axis.

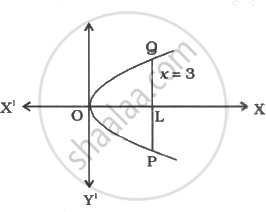
The area of the region bounded by the curve `y^2 = 4x` and the line `x` = 3 is A

= Area of region PQR

= 2 Area of the region OL`theta`

Area below and above `x`-axis are equal.

= `2int_0^3 ydx = 2int_0^3 2sqrt(x)dx`

= `4 2/3(x^(3/2))^3 = 8/3 * 3^(3/2)`

= `8/3 sqrt(27) = 8sqrt(3)` sq.units.