Advertisements
Advertisements
Question
(y − 20)° and (y + 30)° are the measures of complementary angles. Find the measure of each angle.
Solution
The sum of two complementary angles is 90°
∴ (y − 20)° + (y + 30)° = 90°
⇒ y + y + 30 − 20 = 90
⇒ 2y + 10 = 90
⇒ 2y = 90 − 10
⇒ 2y = 80
⇒ y = `80/2`
⇒ y = 40
Measure of first angle = (y – 20)°
= (40 – 20)°
= 20°
Measure of second angle = (y + 30)°
= (40 + 30)°
= 70°
Hence, the measures of the two angles are 20° and 70°.
RELATED QUESTIONS
The measure of the angle is given below. Write the measure of the complementary angle.
55°
Find the measure of the complementary angle of the following angle.
(40 - x)°
What is the measure of the complement of the following angles?
45º
20° and 70° are complementary
Find the supplementary/complementary angle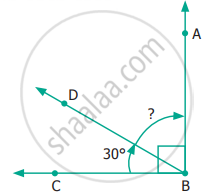
From the figure given below, classify the following pair of angles into complementary and non complementary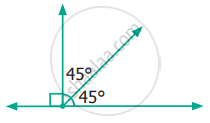
Which angle is equal to twice its complement?
Two complementary angles are in ratio 7 : 2. Find the angles
The difference of two complementary angles is 30°. Then, the angles are ______.
In a pair of complementary angles, each angle cannot be more than ______ .
