Advertisements
Advertisements
प्रश्न
(4, 2) and (-1, 5) are the adjacent vertices ofa parallelogram. (-3, 2) are the coordinates of the points of intersection of its diagonals. Find the coordinates of the other two vertices.
उत्तर

Let the coordinates of C and D be (x, y) and (a , b) respectively.
Midpoint of AC is O coordinates of O are ,
O (-3 , 2) = O `((4 + "x")/2 , (2 + "y")/2)`
`-3 = (4 + "x")/2 , 2 = (2 + "y")/2`
- 6 = 4 + x , 4 = 2 + y
x = - 10 , y = 2
C (-10 , 2)
Similarly , coordinates of midpoint of DB , i.e. O are ,
O (-3 , 2) = O `(("a" - 1)/2 , ("b" + 5)/2)`
`-3 = ("a" - 1)/2 , 2 = ("b" + 5)/2`
- 6 = a - 1 , 4 = b + 5
a = -5 , b = -1
D (- 5 , -1)
Thus , the coordinates of each other two vertices are (-10 , 2) and (-5 - 1)
APPEARS IN
संबंधित प्रश्न
Find the mid-point of the line segment joining the points:
(–6, 7) and (3, 5)
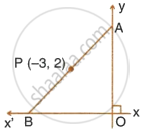
P(–3, 2) is the mid-point of line segment AB as shown in the given figure. Find the co-ordinates of points A and B.
(–5, 2), (3, −6) and (7, 4) are the vertices of a triangle. Find the length of its median through the vertex (3, −6).
A(–1, 0), B(1, 3) and D(3, 5) are the vertices of a parallelogram ABCD. Find the co-ordinates of vertex C.
Find the coordinates of midpoint of the segment joining the points (22, 20) and (0, 16).
The mid point of the line segment joining the points (p, 2) and (3, 6) is (2, q). Find the numerical values of a and b.
As shown in the figure. two concentric circles are given and line AB is the tangent to the smaller circle at T. Shown that T is the midpoint of Seg AB

Find the mid-point of the line segment joining the points
(−2, 3) and (−6, −5)
A(−3, 2), B(3, 2) and C(−3, −2) are the vertices of the right triangle, right angled at A. Show that the mid-point of the hypotenuse is equidistant from the vertices
From the figure given alongside, find the length of the median AD of triangle ABC. Complete the activity.
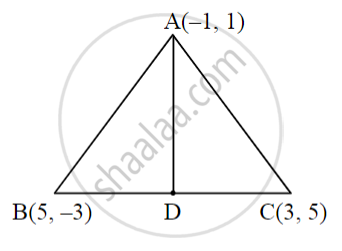
Solution:
Here A(–1, 1), B(5, – 3), C(3, 5) and suppose D(x, y) are coordinates of point D.
Using midpoint formula,
x = `(5 + 3)/2`
∴ x = `square`
y = `(-3 + 5)/2`
∴ y = `square`
Using distance formula,
∴ AD = `sqrt((4 - square)^2 + (1 - 1)^2`
∴ AD = `sqrt((square)^2 + (0)^2`
∴ AD = `sqrt(square)`
∴ The length of median AD = `square`
