Advertisements
Advertisements
प्रश्न
A chord of length 24 cm is at a distance of 5 cm from the center of the circle. Find the length of the chord of the same circle which is at a distance of 12 cm from the center.
उत्तर
Let AB be the chord of length 24 cm and O be the center of the circle.
Let OC be the perpendicular drawn from O to AB.
We know, that the perpendicular to a chord, from the center of a circle, bisects the chord.
∴ AC = CB = 12 cm
In OCA,
OA2 = OC2 + AC2 ....( By Pythagoras theorem )
=(5)2 + ( 12 )2 = 169
⇒ OA = 13 cm
∴ radius of the circle = 13 cm.
Let A ' B ' be the new chord at a distance of 12 cm from the center.
∴ ( OA' )2 = ( OC' )2 + ( A'C' )2
⇒ ( A'C' )2 = ( 13 )2 - ( 12 )2 = 25
∴ A'C' = 5 cm
Hence, length of the new chord = 2 x 5 = 10 cm.
APPEARS IN
संबंधित प्रश्न
A chord of length 8 cm is drawn at a distance of 3 cm from the centre of a circle. Calculate the radius of the circle.
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords, if both the chords are
(i) on the opposite sides of the centre,
(ii) on the same side of the centre.
A chord CD of a circle whose centre is O, is bisected at P by a diameter AB.
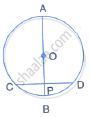
Given OA = OB = 15 cm and OP = 9 cm. calculate the length of:
(i) CD (ii) AD (iii) CB
A chord CD of a circle whose center is O is bisected at P by a diameter AB. Given OA = OB = 15 cm and OP = 9 cm.
Calculate the lengths of: (i) CD ; (ii) AD ; (iii) CB.
In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. Find the distance between the chords,
if both the chords are:
(i) on the opposite sides of the centre;
(ii) on the same side of the centre.
The figure shows two concentric circles and AD is a chord of a larger circle.
Prove that: AB = CD.
In the following figure; P and Q are the points of intersection of two circles with centers O and O'. If straight lines APB and CQD are parallel to OO';
prove that: (i) OO' = `1/2`AB ; (ii) AB = CD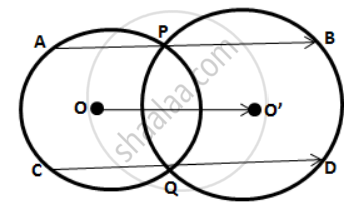
In the given figure, O is the center of the circle with radius 20 cm and OD is perpendicular to AB. If AB = 32 cm,
find the length of CD.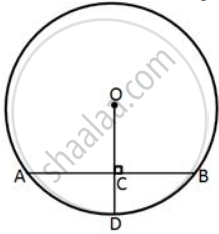
In the given figure, l is a line intersecting the two concentric circles, whose common center is O, at the points A, B, C, and D. Show that AB = CD.
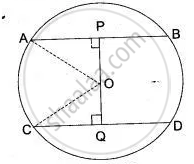
In Fig. O is the centre of the circle with radius 5 cm. OP⊥ AB, OQ ⊥ CD, AB || CD, AB = 8 cm and CD = 6 cm. Determine PQ.