Advertisements
Advertisements
प्रश्न
A flywheel of mass 8 kg and radius 10 cm rotating with a uniform angular speed of 5 rad/sec about its axis of rotation, is subjected to an accelerating torque of 0.01 Nm for 10 seconds. Calculate the change in its angular momentum and change in its kinetic energy.
उत्तर
Given:
M = 8 kg, R = 10 cm = 0.1 m,
ω1 = 5 rad/s, τ = 0.01 Nm, t = 10 s
To find:
- Change in angular momentum (∆L)
- Change in K.E. (∆K.E.)
Formulae:
- I = `"MR"^2/2`
- τ = `I((ω_2 - ω_1)/t)`
- ∆L = I(ω2 - ω1)
- ∆K.E. = `1/2I(ω_2^2 - ω_1^2)`
Calculation:
From formula (i),
I = `(8 xx (0.1)^2)/2`
= 0.04 kgm2
From formula (ii),
ω2 = `((tau xx t))/I + ω_1`
= `(0.01 xx 10)/0.04 + 5`
= 7.5 rad/s
From formula (iii),
∆L = 0.04(7.5 – 5) = 0.1 kg m2/s
From formula (iv),
∆K.E. = `1/2 xx 0.04 xx (7.5^2 - 5^2)`
= 0.625 J
The change in its angular momentum and change in its kinetic energy is 0.1 kg m2/s and 0.625 J respectively.
संबंधित प्रश्न
Obtain an expression for the torque acting on a rotating body with constant angular acceleration. Hence state the dimensions and SI unit of torque.
A stone is tied to one end of a string. Holding the other end, the string is whirled in a horizontal plane with progressively increasing speed. It breaks at some speed because ______
A 500 kg car takes a round turn of the radius of 50m with a velocity of 36 km/hr. The centripetal force is ______.
A flywheel is revolving with a constant angular velocity. A chip of its rim breaks and flies away. What will be the effect on its angular velocity?
An electron(e) is revolving in a circular orbit of radius r in the hydrogen atom. The angular momentum of the electron is (M = magnetic dipole moment associated with it and m = mass of electron)
A stone of mass 1 kg is rotated in a horizontal circle of radius 0.5 m. If it makes `100/pi` rps, then its angular momentum is ______
A charged particle (charge = q: mass = m) is rotating in a circle of radius 'R' with uniform speed 'v'. The ratio of its magnetic moment (M) to the angular momentum (L) is ______
Angular momentum of the earth revolving around the sun is proportional to rn , where r is the distance between the earth and the sun. Value of n is ____________.
If the angular momentum of a body increases by 50%, then its kinetic energy of rotation increases by ______ (M.I. remains constant)
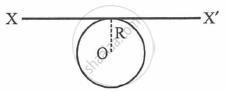
A thin metal wire of length 'L' and uniform linear mass density 'ρ' is bent into a circular coil with 'O' as centre. The moment of inertia of a coil about the axis XX' is ______.
If the kinetic energy of rotation of a body is doubled, then its angular momentum ____________.
The ratio of the dimensions of Planck's constant to that of moment of inertia is the dimensions of ______.
A homogeneous disc of mass 2 kg and radius 15 cm is rotating about its axis (which is fixed) with an angular velocity of 4 radian/s. The linear momentum of the disc is ____________.
mass is whirled in a circular path with constant angular velocity and its linear velocity is v. If the string is now halved keeping the angular momentum same, the linear velocity is ______.
An electron has a mass of 9.1 x 10-31 kg. It revolves round the nucleus in a circular orbit of radius 0.529 x 10-10 metre at a speed of 2.2 x 106 m/s. The magnitude of its linear momentum in this motion is ____________.
The direction of angular momentum of particle is ____________.
An electron of mass 'm' revolving around the nucleus in a circular orbit of radius 'r' has angular momentum 'L'. The magnetic field produced by the electron at the centre of the orbit is e = electric charge, µ0 = permeability of free space ____________.
A particle is revolving in anticlockwise sense along the circumference of a circle of radius 'r' with linear velocity 'v', then the angle between 'v' and angular velocity 'ω' will be ______.
lf 'I' is the moment of inertia and 'L' is angular momentum of a rotating body, then `L^2/(2I)` is its ______.
Three-point masses each of mass 'M' are placed at the corners of an equilateral triangle of side 'a'. The moment of inertia of this system about an axis passing through one side of a triangle is ______.
A body is rotating about its own axis. Its rotational kinetic energy is x and its angular momentum is y, hence its moment of inertia about the axis is ______.
The difference in the angular momentum of an electron in two successive orbits of a hydrogen atom is ______.
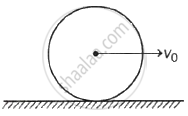
A sphere rolls without slipping on a rough horizontal surface with centre of mass speed v0. If mass of the sphere is M and its radius is R, then what is the angular momentum of the sphere about the point of contact?
The angular momentum of the electron in the second orbit of hydrogen atom is L. The angular momentum in the third orbit is ______.
Define moment of inertia.
