Advertisements
Advertisements
प्रश्न
A jet airplane travelling at the speed of 500 km h–1 ejects its products of combustion at the speed of 1500 km h–1 relative to the jet plane. What is the speed of the latter with respect to an observer on ground?
उत्तर १
Speed of the jet airplane, vjet = 500 km/h
Relative speed of its products of combustion with respect to the plane,
vsmoke = – 1500 km/h
Speed of its products of combustion with respect to the ground = v′smoke
Relative speed of its products of combustion with respect to the airplane,
vsmoke = v′smoke – vjet
– 1500 = v′smoke – 500
v′smoke = – 1000 km/h
The negative sign indicates that the direction of its products of combustion is opposite to the direction of motion of the jet airplane.
उत्तर २
Velocity of jet airplane w.r.t observer on ground = 500 km/h.
If Vj and v0 represent the velocities of jet and observer respectively, then vj – vo = 500 km h-1
Similarly, if vc represents the velocity of the combustion products w.r.t jet plane, then vc – vg = -1500 km/h
The negative sign indicates that the combustion products move in a direction opposite to that of jet.
Speed of combustion products w.r.t. observer
= vc – u0 = (vc – vj) + (vj – v0) = (-1500 + 500) km h-1 = -1000 km h-1.
APPEARS IN
संबंधित प्रश्न
A woman starts from her home at 9.00 am, walks with a speed of 5 km h–1 on a straight road up to her office 2.5 km away, stays at the office up to 5.00 pm, and returns home by an auto with a speed of 25 km h–1. Choose suitable scales and plot the x-t graph of her motion.
Read the statement below carefully and state, with reason and example, if it is true or false:
A particle in one-dimensional motion with zero speed may have non-zero velocity.
In Exercise, we have carefully distinguished between average speed and magnitude of average velocity. No such distinction is necessary when we consider instantaneous speed and magnitude of velocity. The instantaneous speed is always equal to the magnitude of instantaneous velocity. Why?
Suggest a suitable physical situation for the following graph:

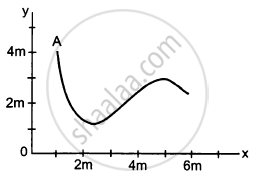
Figure A particle starts from a point A and travels along the solid curve shown in the following figure. Find approximately the position B of the particle such that the average velocity between the position A and B has the same direction as the instantaneous velocity at B.
Two cars are moving with speeds of 40 km/hr due North and 40 km/hr due South respectively. Do they have the same velocity?
In one dimensional motion, instantaneous speed v satisfies 0 ≤ v < v0.
A particle executes the motion described by x(t) = x0 (1 − e − γt); t ≥ 0, x0 > 0. Where does the particle start and with what velocity?
A particle executes the motion described by x(t) = x0(1 − e −γt); t ≥ 0, x0 > 0. Find maximum and minimum values of x(t), v(t), a(t). Show that x(t) and a(t) increase with time and v(t) decreases with time.
A monkey climbs up a slippery pole for 3 seconds and subsequently slips for 3 seconds. Its velocity at time t is given by v(t) = 2t (3 – t); 0 < t < 3 and v(t) = – (t – 3)(6 – t) for 3 < t < 6 s in m/s. It repeats this cycle till it reaches the height of 20 m.
- At what time is its velocity maximum?
- At what time is its average velocity maximum?
- At what time is its acceleration maximum in magnitude?
- How many cycles (counting fractions) are required to reach the top?
