Advertisements
Advertisements
प्रश्न
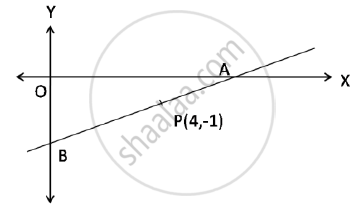
A line AB meets X – axis at A and Y –axis at B. P (4, -1) divides AB in the ratio 1 : 2.
1) Find the coordinates of A and B.
2) Find the equation of the line through P and perpendicular to AB.
उत्तर
1) Since A lies on the x-axis, let the coordinates of A be (x, 0).
Since B lies on the y-axis, let the coordinates of B be (0, y).
Let m = 1 and n = 2.
Using section formula
Coordinates of P = `((1(0)+2(x))/(1+2), (1y+2(0))/(1+2))`
`=> (4, 1) = ("2x"/3, y/3)`
`=> (2x)/3 = 4 and y/3 = -1`
=> x = 6 and y = -3.
So, the coordinates of A are (6,0) and that of B are (0, -3).
2) Slope of AB = `(-3-0)/(0-6) = (-3)/(-6) = 1/2`
=> Slope of line perpendicular to AB=m= -2
P = (4,-1)
⇒Required equation is
`y - y_1 = m(x - x_1)`
`=> y - (-1) = -2(x - 4)`
`=> y + 1 = -2x + 8`
`=> 2x + y = 7`
APPEARS IN
संबंधित प्रश्न
The line y = mx + 8 contains the point (−4, 4), calculate the value of m.
The co-ordinates of two points A and B are (-3, 4) and (2, -1) Find: the co-ordinates of the point where the line AB intersects the y-axis.
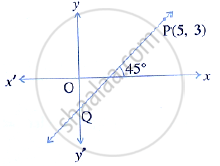
The line through P (5, 3) intersects y-axis at Q.
Find the co-ordinates of Q.
Write down the equation of the line whose gradient is `-2/5` and which passes through point P, where P divides the line segement joining A(4, −8) and B(12, 0) in the ratio 3 : 1.
A(7, −1), B(4, 1) and C(−3, 4) are the vertices of a triangle ABC. Find the equation of a line through the vertex B and the point P in AC; such that AP : CP = 2 : 3.
Find the value of a if the line 4 x = 11 - 3y passes through the point (a, 5)
Find the inclination of a line whose gradient is 5.6713
Find the inclination of a line whose gradient is 0.5317
Find the equation of a line that has Y-intercept 3 units and is perpendicular to the line joining (2, – 3) and (4, 2).
Find the value of ‘a’ for which the following points A (a, 3), B (2, 1) and C (5, a) are collinear. Hence find the equation of the line.
