Question

Find the value of ‘a’ for which the following points A (a, 3), B (2, 1) and C (5, a) are collinear. Hence find the equation of the line.

Answer 1

Equation of the line passing through AC is
(y - 3) = `((a - 3)/(5 - a))(x - a)`
As if A, B and C are callinear than B will satisfy it, i.e.,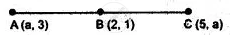

(1 - 3) = `((a - 3)/(5 - a)) (2 - a)`
-2(5 - a) = (a - 3) (2 - a)
-10 + 2a = 2a - 6 - a² + 3a
a² - 3a - 4 = 0
a² - 4a +a - 4 = 0
a(a - 4) + 1 (a - 4) = 0
(a - 4) (a + 1) = 0
⇒ a = 4 or -1.
Thus, required equation of straight line is
(y - 3) = `((4 - 3)/(5 - 4))(x - 4)`
y - 3 = `(1/1)(x - 4)`
x - y - 1 = 0
or
(y - 3) = `((-1 - 3)/(5 + 1))(x + 1)`
(y - 3) = `(-4/6)(x + 1)`
y - 3 = `(-2)/(3)(x + 1)`
3y - 9 - 2x - 2
2x + 3y - 7 = 0.