Advertisements
Advertisements
प्रश्न
If the image of the point (2,1) with respect to the line mirror be (5, 2). Find the equation of the mirror.
उत्तर
Let CD be the line mirror with slope m1.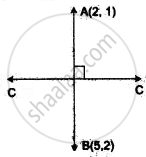
Now the slope of the line joining A(2, 1) and B(5, 2).
m2 = `(2 - 1)/(5 - 2) = (1)/(3)`
Since CD ⊥ AB
So, m1m2 = -1
⇒ `m_1 xx (1)/(3)` = -1
⇒ m1 = -3.
Now mid point of AB = `((2+ 5)/2, (1 + 2)/2) = (7/2, 3/2)`
Equation of the mirror CD,
y = y1 = m(x - x1)
⇒ `y - (3)/(2) = -3(x-7/2)`
⇒ `y - (3)/(2) = -3x + (21)/(2)`
⇒ 2y - 3 = -6x + 21
⇒ 6x + 2y - 3 - 21 = 0
⇒ 6x + 2y - 24 = 0
or 3x + y - 12 = 0.
APPEARS IN
संबंधित प्रश्न
The line y = 3x – 2 bisects the join of (a, 3) and (2, −5), find the value of a.
Find the equation of the line whose slope is `-4/3` and which passes through (−3, 4).
The line through P (5, 3) intersects y-axis at Q.
write the equation of the line
A(1, 4), B(3, 2) and C(7, 5) are vertices of a triangle ABC. Find the co-ordinates of the centroid of triangle ABC.
The line segment joining the points A(3, −4) and B(−2, 1) is divided in the ratio 1 : 3 at point P in it. Find the co-ordinates of P. Also, find the equation of the line through P and perpendicular to the line 5x – 3y = 4.
A line intersects x-axis at point (−2, 0) and cuts off an intercept of 3 units from the positive side of y-axis. Find the equation of the line.
Given equation of line L1 is y = 4.
- Write the slope of line L2 if L2 is the bisector of angle O.
- Write the co-ordinates of point P.
- Find the equation of L2.
Find the value of a line parallel to the following line:
`"x"/4 +"y"/3` = 1
Find the equation of a line passing through the intersection of x + 2y + 1= 0 and 2x - 3y = 12 and perpendicular to the line 2x + 3y = 9
Given equation of line L1 is y = 4.
(i) Write the slope of line, if L2 is the bisector of angle O.
(ii) Write the coordinates of point P.
(iii) Find the equation of L2