Advertisements
Advertisements
प्रश्न
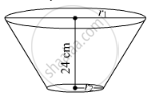
A metallic bucket, open at the top, of height 24 cm is in the form of the frustum of a cone, the radii of whose lower and upper circular ends are 7 cm and 14 cm, respectively. Find
- the volume of water which can completely fill the bucket;
- the area of the metal sheet used to make the bucket.
उत्तर
We have,
Height, h = 24 cm,
Upper base radius, R = 14 cm and lower base radius, r = 7 cm
Also, the slant height, `l =sqrt(("R"-"r")^2 + "h"^2)`
`= sqrt((14-7)^2+24^2)`
`=sqrt(7^2+24^2)`
`=sqrt(49+576)`
`= sqrt(625)`
= 25 cm
i . Volume of the bucket`= 1/3 pi"h"("R"^2+"r"^2+"Rr")`
`= 1/3xx22/7xx24xx(14^2+7^2+14+7)`
`=22/7xx8xx(196+49+98)`
`=22/7xx343`
= 8624 cm3
So, the volume of water which can completely fill the bucket is 8624 cm3
ii . surface area of the bucket = π (R + r)l + πr2
`=22/7xx(14+7)xx25+22/7xx7xx7`
`=22/7xx21xx25+22xx7`
= 22 × 3 × 25 + 22 × 7
= 1650 + 154
= 1804 cm2
So, the area of the metal sheet used to make the bucket is 1804 cm2 .
APPEARS IN
संबंधित प्रश्न
A 5 m wide cloth is used to make a conical tent of base diameter 14 m and height 24 m. Find the cost of cloth used at the rate of Rs 25 per metre ?\[[Use \pi = \frac{22}{7}]\]
The circumferences of circular faces of a frustum are 132 cm and 88 cm and its height is 24 cm. To find the curved surface area of the frustum complete the following activity.( \[\pi = \frac{22}{7}\])
The surface area of a sphere is the same as the curved surface area of a cone having the radius of the base as 120 cm and height 160 cm. Find the radius of the sphere.
The radii of the ends of a frustum of a right circular cone are 5 metres and 8 metres and its lateral height is 5 metres. Find the lateral surface and volume of the frustum.
A cylinder with base radius of 8 cm and height of 2 cm is melted to form a cone of height 6 cm. The radius of the cone is
The perimeters of the two circular ends of a frustum of a cone are 48 cm and 36 cm. If the height of the frustum is 11 cm, then find its volume and curved surface area.
A frustum of a right circular cone is of height 16 cm with radius of its ends as 8 cm and 20 cm. Then, the volume of the frustum is
By melting a solid sphere of radius 5 cm a solid right circular cone of the same circular base radius is made. The height of cone is ______.
A cylinder and a cone area of same base radius and of same height. The ratio of the volume of cylinder to that of cone is ______.
If radius of the base of cone is 7 cm and height is 24 cm, then find its slant height.
