Advertisements
Online Mock Tests
Chapters
2: Polynomials
3: Linear Equations in two variables
4: Triangles
5: Trigonometric Ratios
6: T-Ratios of some particular angles
7: Trigonometric Ratios of Complementary Angles
8: Trigonometric Identities
9: Mean, Median, Mode of Grouped Data, Cumulative Frequency Graph and Ogive
10: Quadratic Equations
11: Arithmetic Progression
12: Circles
13: Constructions
14: Height and Distance
15: Probability
16: Coordinate Geomentry
17: Perimeter and Areas of Plane Figures
18: Area of Circle, Sector and Segment
▶ 19: Volume and Surface Area of Solids
![RS Aggarwal solutions for Mathematics [English] Class 10 chapter 19 - Volume and Surface Area of Solids RS Aggarwal solutions for Mathematics [English] Class 10 chapter 19 - Volume and Surface Area of Solids - Shaalaa.com](/images/9789350271810-mathematics-english-class-10_6:362518cae02c41f896f78fdfb22bac39.jpg)
Advertisements
Solutions for Chapter 19: Volume and Surface Area of Solids
Below listed, you can find solutions for Chapter 19 of CBSE RS Aggarwal for Mathematics [English] Class 10.
RS Aggarwal solutions for Mathematics [English] Class 10 19 Volume and Surface Area of Solids Exercise 19A [Pages 874 - 877]
Two cubes each of volume 27 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
The volume of a hemisphere is 2425 `1/2` cm3 . Find its curved surface area.
If the total surface area of a solid hemisphere is 462 cm2, then find its volume.
A 5-m-wide cloth is used to make a conical tent of base diameter 14 m and height 24 m. Find the cost of cloth used at the rate of ₹25 per metre.
If the volumes of two cones are in the ratio of 1:4 and their diameters are in the ratio of 4:5, then find the ratio of their heights.
The slant height of a conical mountain is 2.5 km and the area of its base is 1.54 km2. Find the height of the mountain.
The sum of the radius of the base and the height of a solid cylinder is 37 metres. If the total surface area of the cylinder be 1628 sq metres, then find its volume.
The surface area of a sphere is 2464 cm2. If its radius be doubled, then what will be the surface area of the new sphere?
A military tent of height 8.25 m is in the form of a right circular cylinder of base diameter 30 m and height 5.5 m surmounted by a right circular cone of same base radius. Find the length of canvas used in making the tent, if the breadth of the canvas is 1.5 m.
A tent is in the shape of a right circular cylinder up to a height of 3 m and conical above it. The total height of the tent is 13.5 m and the radius of its base is 14 m. Find the cost of cloth required to make the tent at the rate of Rs 80 per square metre.
A circus tent is cylindrical to a height of 3 m and conical above it. If its base radius is 52.5 m and the slant height of the conical portion is 53 m, find the area of canvas needed to make the tent.
A rocket is in the form of a circular cylinder closed at the lower end and a cone of the same radius is attached to the top. The radius of the cylinder is 2.5 m, its height is 21 m and the slant height of the cone is 8 m. Calculate the total surface area of the rocket.
A solid is in the shape of a cone surmounted on a hemisphere, the radius of each of them being 3.5 cm and the total height of the solid is 9.5 cm. Find the volume of the solid.
A toy is in the form of a cone mounted on a hemisphere of common base radius 7 cm. The total height of the toy is 31 cm. Find the total surface area of the toy.
A toy is in the shape of a cone mounted on a hemisphere of same base radius. If the volume of the toy is 231 cm3 and its diameter is 7 cm, then find the height of the toy.
A cylindrical container of radius 6 cm and height 15 cm is filled with ice-cream. The whole ice-cream has to be distributed to 10 children in equal cones with hemispherical tops. If the height of the conical portion is 4 times the radius of its base, then find the radius of the ice-cream cone.
A vessel is in the form of a hemispherical bowl surmounted by a hollow cylinder. The diameter of the hemisphere is 21 cm and the total height of the vessel is 14.5 cm. Find its capacity.
A toy is in the form of a cylinder with hemispherical ends. If the whole length of the toy is 90 cm and its diameter is 42 cm, then find the cost of painting the toy at the rate of 70 paise per sq cm.
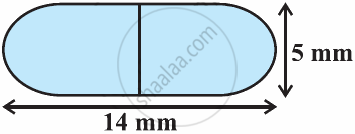
A medicine capsule is in the shape of cylinder with two hemispheres stuck to each of its ends (see the given figure). The length of the entire capsule is 14 mm and the diameter of the capsule is 5 mm. Find its surface area. [Use π = `22/7`]
A solid is composed of a cylinder with hemispherical ends. If the whole length of the solid is 98 cm and the diameter of each of its hemispherical ends is 28 cm, find the cost of polishing the surface of the solid at the rate of 15 paise per sq cm.
A solid is in the form of a right circular cone mounted on a hemisphere. The radius of the hemisphere is 2.1 cm and the height of the cone is 4 cm. The solid is placed in a cylindrical tub full of water in such a way that the whole solid is submerged in water. If the radius of the cylinder is 5 cm and its height is 9.8 cm, find the volume of the water left in the tub.
From a solid cylinder whose height is 8 cm and radius 6 cm, a conical cavity of height 8 cm and base radius 6 cm is hollowed out. Find the volume of the remaining solid. Also, find the total surface area of the remaining solid.
From a solid cylinder of height 2.8 cm and diameter 4.2 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid.
From a solid cylinder of height 14 cm and base diameter 7 cm, two equal conical holes each of radius 2.1 cm and height 4 cm are cut off. Find the volume of the remaining solid.
A metallic cylinder has radius 3 cm and height 5 cm. To reduce its weight, a conical hole is drilled in the cylinder. The conical hole has a radius of `3/2` cm and its depth is `8/9` cm. Calculate the ratio of the volume of metal left in the cylinder to the volume of metal taken out in conical shape.
A spherical glass vessel has a cylindrical neck that is 7 cm long and 4 cm in diameter. The diameter of the spherical part is 21 cm. Find the quantity of water the vessel can hold.
The adjoining figure represents a solid consisting of a cylinder surmounted by a cone at one end and a hemisphere at the other. Find the volume of the solid.

From a cubical piece of wood of side 21 cm, a hemisphere is carved out in such a way that the diameter of the hemisphere is equal to the side of the cubical piece. Find the surface area and volume of the remaining piece.
A cubical block of side 10 cm is surmounted by a hemisphere. What is the largest diameter that the hemisphere can have? Find the cost of painting the total surface area of the solid so formed, at the rate of ₹5 per 100 sq cm. [Use ππ = 3.14]
A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The radius and height of the cylindrical part are 5 cm and 13 cm, respectively. The radii of the hemispherical and the conical parts are the same as that of the cylindrical part. Find the surface area of the toy, if the total height of the toy is 30 cm.
The inner diameter of a glass is 7 cm and it has a raised portion in the bottom in the shape of a hemisphere, as shown in the figure. If the height of the glass is 16 cm, find the apparent capacity and the actual capacity of the glass.

A wooden toy is in the shape of a cone mounted on a cylinder, as shown in the figure. The total height of the toy is 26 cm, while the height of the conical part is 6 cm. The diameter of the base of the conical part is 5 cm and that of the cylindrical part is 4 cm. The conical part and the cylindrical part are respectively painted red and white. Find the area to be painted by each of these colours. `["Take" pi = 22/7]`

RS Aggarwal solutions for Mathematics [English] Class 10 19 Volume and Surface Area of Solids Exercise 19B [Pages 897 - 900]
The dimensions of a metallic cuboid are 100cm× 80 cm× 64cm. It is melted and recast into a cube. Find the surface area of the cube.
A cone of height 20 cm and radius of base 5 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the diameter of the sphere.
Metallic spheres of radii 6 cm, 8 cm and 10 cm, respectively are melted to form a single solid sphere. Find the radius of the resulting sphere.
A solid metal cone with base radius of 12 cm and height 24 cm is melted to form solid spherical balls of diameter 6 cm each. Find the number of balls thus formed.
The radii of internal and external surfaces of a hollow spherical shell are 3 cm and 5 cm, respectively. It is melted and recast into a solid cylinder of diameter 14 cm. Find the height of the cylinder.
The internal and external diameters of a hollow hemispherical shell are 6 cm and 10 cm, respectively. It is melted and recast into a solid cone of base diameter 14 cm. Find the height of the cone so formed.
A copper rod of diameter 2 cm and length 10 cm is drawn into a wire of uniform thickness and length 10 m. Find the thickness of the wire.
A hemispherical bowl of internal diameter 30 cm contains some liquid. This liquid is to be poured into cylindrical bottles of diameter 5 cm and height 6 cm each. Find the number of bottles required.
A solid metallic sphere of diameter 21 cm is melted and recast into a number of smaller cones, each of diameter 3.5 cm and height 3 cm. Find the number of cones so formed.
A spherical cannon ball, 28 cm in diameter, is melted and recast into a right circular conical mould with base diameter of 35 cm. Find the height of the cone.
A spherical ball of radius 3 cm is melted and recast into three spherical balls. The radii of two of these balls are 1.5 cm and 2 cm. Find the radius of the third ball.
A spherical shell of lead, whose external and internal diameters are 24 cm and 18 cm, is melted and recast into a right circular cylinder 37 cm high. Find the diameter of the base of the cylinder.
A hemisphere of lead of radius 9 cm is cast into a right circular cone of height 72 cm. Find the radius of the base of the cone.
A spherical ball of diameter 21 cm is melted and recast into cubes, each of side 1 cm. Find the number of cubes so formed.
How many lead balls, each of radius 1 cm, can be made from a sphere of radius 8 cm?
A solid sphere of radius 3 cm is melted and then cast into small spherical balls, each of diameter 0.6 cm. Find the number of balls obtained.
The diameter of a sphere is 42 cm. It is melted and drawn into a cylindrical wire of diameter 2.8 cm. Find the length of the wire.
The diameter of a copper sphere is 18 cm. It is melted and drawn into a long wire of uniform cross-section. If the length of the wire is 108 m, find its diameter.
A hemispherical bowl of internal radius 9 cm is full of water. Its contents are emptied into a cylindrical vessel of internal radius 6 cm. Find the height of water in the cylindrical vessel.
A hemispherical tank, full of water, is emptied by a pipe at the rate of `25/7` litres per second. How much time will it take to empty half the tank if the diameter of the base of the tank is 3 m?
The rain water from a roof of 44 m × 20 m drains into a cylindrical tank having diameter of base 4 m and height 3.5 m. If the tank is just full, then find the rainfall in cm.
The rain water from a 22 m × 20 m roof drains into a cylindrical vessel of diameter 2 m and height 3.5 m. If the rain water collected from the roof fills `4/5` th of the cylindrical vessel, then find the rainfall in centimetre.
A solid right circular cone of height 60 cm and radius 30 cm is dropped in a right circular cylinder full of water, of height 180 cm and radius 60 cm. Find the volume of water left in the cylinder, in cubic metres.
Water is flowing through a cylindrical pipe of internal diameter 2 cm, into a cylindrical tank of base radius 40 cm, at the rate of 0.4 m per second. Determine the rise in level of water in the tank in half an hour.
Water is flowing at the rate of 6 km/hr through a pipe of diameter 14 cm into a rectangular tank which is 60 m long and 22 m wide. Determine the time in which the level of water in the tank will rise by 7 cm.
Water in a canal, 6 m wide and 1.5 m deep, is flowing at a speed of 4 km/hr. How much area will it irrigate in 10 minutes if 8 cm of standing water is needed for irrigation?
A farmer connects a pipe of internal diameter 25 cm from a canal into a cylindrical tank in his field, which is 12 m in diameter and 2.5 m deep. If water flows through the pipe at the rate of 3.6 km/hr, then in how much time will the tank be filled? Also, find the cost of water if the canal department charges at the rate of ₹ 0.07 per m3.
150 spherical marbles, each of diameter 1.4 cm, are dropped in a cylindrical vessel of diameter 7 cm containing some water, which are completely immersed in water. Find the rise in the level of water in the vessel.
Marbles of diameter 1.4 cm are dropped into a cylindrical beaker of diameter 7 cm, containing some water. Find the number of marbles that should be dropped into the beaker so that the water level rises by 5.6 cm.
In a village, a well with 10 m inside diameter, is dug 14 m deep. Earth taken out of it is spread all around to a width 5 m to form an embankment. Find the height of the embankment. What value of the villagers is reflected here?
In a corner of a rectangular field with dimensions 35m × 22 m, a well with 14 m inside diameter is dug 8 m deep. The earth dug out is spread evenly over the remaining part of the field. Find the rise in the level of the field.
A copper wire of diameter 6 mm is evenly wrapped on a cylinder of length 18 cm and diameter 49 cm to cover its whole surface. Find the length and the volume of the wire. If the density of the copper be 8.8 g per cm3, then find the weight of the wire.
A right triangle whose sides are 15 cm and 20 cm (other than hypotenuse), is made to revolve about its hypotenuse. Find the volume and surface area of the double cone so formed. (Choose value of π as found appropriate)
A right triangle whose sides are 15 cm and 20 cm (other than hypotenuse), is made to revolve about its hypotenuse. Find the volume and surface area of the double cone so formed. (Choose value of π as found appropriate)
RS Aggarwal solutions for Mathematics [English] Class 10 19 Volume and Surface Area of Solids Exercise 19C [Pages 910 - 912]
A drinking glass is in the shape of a frustum of a cone of height 14 cm. The diameters of its two circular ends are 16 cm and 12 cm. Find the capacity of the glass.
The radii of the circular ends of a solid frustum of a cone are 18 cm and 12 cm and its height is 8 cm. Find its total surface area. [Use π = 3.14]
A metallic bucket, open at the top, of height 24 cm is in the form of the frustum of a cone, the radii of whose lower and upper circular ends are 7 cm and 14 cm, respectively. Find
- the volume of water which can completely fill the bucket;
- the area of the metal sheet used to make the bucket.
A container, open at the top, is in the form of a frustum of a cone of height 24 cm with radii of its lower and upper circular ends as 8 cm and 20 cm, respectively. Find the cost of milk which can completely fill the container at the rate of ₹21 per litre.
A container, open at the top and made up of metal sheet, is in the form of a frustum of a cone of height 16 cm with diameters of its lower and upper ends as 16 cm and 40 cm, respectively. Find the cost of metal sheet used to make the container, if it costs ₹10 per 100 cm2
The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm, and its slant height is 10 cm. Find its capacity and total surface area.
A bucket is in the form of a frustum of a cone. Its depth is 15 cm and the diameters of the top and the bottom are 56 cm and 42 cm, respectively. Find how many litres of water can the bucket hold.
A bucket made up of a metal sheet is in the form of a frustum of a cone of height 16 cm and radii of its lower and upper ends are 8 cm and 20 cm, respectively. Find the cost of the bucket if the cost of metal sheet used is Rs 15 per 100 cm2.
A bucket made up of a metal sheet is in the form of frustum of a cone. Its depth is 24 cm and the diameters of the top and bottom are 30 cm and 10 cm, respectively. Find the cost of completely filling the bucket with milk at the rate of Rs 20 per litre and the cost of metal sheet used if it costs Rs 10 per 100 cm2.
A container in the shape of a frustum of a cone having diameters of its two circular faces as 35 cm and 30 cm and vertical height 14 cm,
is completely filled with oil. If each cm3 of oil has mass 1.2 g, then find the cost of oil in the container if it costs ₹40 per kg.
A bucket is in the form of a frustum of a cone and it can hold 28.49 litres of water. If the radii of its circular ends are 28 cm and 21 cm, then find the height of the bucket.
The radii of the circular ends of a bucket of height 15 cm are 14 cm and r cm (r < 14). If the volume of bucket is 5390 cm3, then find the value of r.
The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm and its slant height is 10 cm. Find its total surface area. [Use π = 3.14.]
A tent is made in the form of a frustum of a cone surmounted by another cone. The diameters of the base and the top of the frustum are 20 m and 6 m, respectively, and the height is 24 m. If the height of the tent is 28 m and the radius of the conical part is equal to the radius of the top of the frustum, find the quantity of canvas required.
A tent consists of a frustum of a cone, surmounted by a cone. If the diameter of the upper and lower circular ends of the frustum be 14 m and 26 m, respectively, the height of the frustum be 8 m and the slant height of the surmounted conical portion be 12 m, find the area of the canvas required to make the tent. (Assume that the radii of the upper circular end of the frustum and the base of the surmounted conical portion are equal.)
The perimeters of the two circular ends of a frustum of a cone are 48 cm and 36 cm. If the height of the frustum is 11 cm, then find its volume and curved surface area.
A solid cone of base radius 10 cm is cut into two parts through the midpoint of its height, by a plane parallel to its base. Find the ratio of the volumes of the two parts of the cone.
The height of a right circular cone is 20 cm. A small cone is cut off at the top by a plane parallel to the base. If its volume be `1/8` of the volume of the given cone, then at what height above the base is the section made?
A solid metallic right circular cone 20 cm high and whose vertical angle is 60°, is cut into two parts at the middle of its height by a plane parallel to its base. If the frustum so obtained be drawn into a wire of diameter `1/12` cm, then find the length of the wire.
A fez, the cap used by the Turks, is shaped like the frustum of a cone. If its radius on the open side is 10 cm, radius at the upper base is
4 cm and its slant height is 15 cm, then find the area of material used for making it.
An oil funnel made of tin sheet consists of a 10 cm long cylindrical portion attached to a frustum of a cone. If the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, then find the area of the tin sheet required to make the funnel.

RS Aggarwal solutions for Mathematics [English] Class 10 19 Volume and Surface Area of Solids Exercise [Pages 914 - 917]
A river 1.5 m deep and 36 m wide is flowing at the rate of 3.5 km/hr. Find the amount of water (in cubic metres) that runs into the sea per minute.
The volume of a cube is 729 cm3. Find its surface area.
How many cubes of 10 cm edge can be put in a cubical box of 1 m edge?
Three cubes of iron whose edges are 6 cm, 8 cm and 10 cm, respectively are melted and formed into a single cube. Find the edge of the new cube formed.
Five identical cubes, each of edge 5 cm, are placed adjacent to each other. Find the volume of the resulting cuboid.
The volumes of two cubes are in the ratio 8 : 27. Find the ratio of their surface areas.
The volume of a right circular cylinder with its height equal to the radius is `25"1"/7` cm3. Find the height of the cylinder.
The ratio between the radius of the base and the height of a cylinder is 2 : 3. If the volume of the cylinder is 12936 cm3, then find the radius of the base of the cylinder.
The radii of two cylinders are in the ratio of 2 : 3 and their heights are in the ratio of 5 : 3. Find the ratio of their volumes.
66 cubic cm of silver is drawn into a wire 1 mm in diameter. Calculate the length of the wire in metres.
If the area of the base of a right circular cone is 3850 cm2 and its height is 84 cm, then find the slant height of the cone.
A cylinder with base radius 8 cm and height 2 cm is melted to form a cone of height 6 cm. Calculate the radius of the base of the cone.
A right cylindrical vessel is full of water. How many right cones having the same radius and height as those of the right cylinder will be needed to store that water?
The volume of a sphere is 4851 cm3. Find its curved surface area.
The curved surface area of a sphere is 5544 cm2. Find its volume.
The surface areas of two spheres are in the ratio of 4 : 25. Find the ratio of their volumes.
A solid metallic sphere of radius 8 cm is melted and recast into spherical balls each of radius 2 cm. Find the number of spherical balls obtained.
How many lead shots each 3 mm in diameter can be made from a cuboid of dimensions 9 cm × 11 cm × 12 cm ?
A metallic cone of radius 12 cm and height 24 cm is melted and made into spheres of radius 2 cm each. How many spheres are formed?
A hemisphere of lead of radius 6 cm is cast into a right circular cone of height 75 cm. Find the radius of the base of the cone.
A copper sphere of diameter 18 cm is drawn into a wire of diameter 4 mm. Find the length of the wire.
The radii of the circular ends of a frustum of height 6 cm are 14 cm and 6 cm, respectively. Find the slant height of the frustum.
Find the ratio of the volume of a cube to that of a sphere which will fit inside it.
Three metallic cubes whose edges are 3 cm, 4 cm and 5 cm, are melted and recast into a single large cube. Find the edge of the new cube formed.
Find the ratio of the volumes of a cylinder, a cone and a sphere, if each has the same diameter and same height?
Two cubes each of volume 125 cm3 are joined end to end to form a solid. Find the surface area of the resulting cuboid.
Three metallic cubes whose edges are 3 cm, 4 cm and 5 cm, are melted and recast into a single large cube. Find the edge of the new cube formed.
A solid metallic sphere of diameter 8 cm is melted and drawn into a cylindrical wire of uniform width. If the length of the wire is 12 m, then find its width.
A 5-m-wide cloth is used to make a conical tent of base diameter 14 m and height 24 m. Find the cost of cloth used, at the rate of ₹25 per metre.
A wooden toy was made by scooping out a hemisphere of same radius from each end of a solid cylinder. If the height of the cylinder is 10 cm and its base is of radius 3.5 cm, then find the volume of wood in the toy.
Three cubes of a metal whose edges are in the ratio 3 : 4 : 5 are melted and converted into a single cube whose diagonal is `12sqrt(3)` cm. Find the edges of the three cubes.
A hollow sphere of external and internal diameters 8 cm and 4 cm, respectively is melted into a solid cone of base diameter 8 cm. Find the height of the cone.
A bucket of height 24 cm is in the form of frustum of a cone whose circular ends are of diameter 28 cm and 42 cm. Find the cost of milk at the rate of ₹30 per litre, which the bucket can hold.
The interior of a building is in the form of a right circular cylinder of diameter 4.2 m and height 4 m surmounted by a cone of same diameter.
The height of the cone is 2.8 m. Find the outer surface area of the building.
A metallic solid right circular cone is of height 84 cm and the radius of its base is 21 cm. It is melted and recast into a solid sphere. Find the
diameter of the sphere.
A toy is in the form of a cone of radius 3.5 cm mounted on a hemisphere of same radius. The total height of the toy is 15.5 cm. Find the total
surface area of the toy.
If the radii of the circular ends of a bucket 28 cm high, are 28 cm and 7 cm, then find its capacity and total surface area.
A bucket is in the form of a frustum of a cone with a capacity of 12308.8 cm3 of water. The radii of the top and bottom circular ends are
20 cm and 12 cm, respectively. Find the height of the bucket. [Use π = 3.14]
A milk container is made of metal sheet in the shape of frustum of a cone whose volume is `"10459" 3/7 "cm"`. The radii of its lower and upper circular ends are 8 cm and 20 cm, respectively. Find the cost of metal sheet used in making the container at the rate of ₹1.40 per cm2.
A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter `"4"2/3` cm and height 3 cm. Find the number of cones so formed.
A cylindrical vessel with internal diameter 10 cm and height 10.5 cm is full of water. A solid cone of base diameter 7 cm and height 6 cm is completely immersed in water. Find the volume of water
- displaced out of the cylinder
- left in the cylinder.
RS Aggarwal solutions for Mathematics [English] Class 10 19 Volume and Surface Area of Solids Multiple Choice Questions [Pages 917 - 927]
A cylindrical pencil sharpened at one end is a combination of
a cylinder and a cone
a cylinder and frustum of a cone
a cylinder and a hemisphere
two cylinders
A shuttlecock used for playing badminton is a combination of

cylinder and a hemisphere
frustum of a cone and a hemisphere
a cone and a hemisphere
a cylinder and a sphere
A funnel is a combination of
a cylinder and a cone
a cylinder and a hemisphere
a cylinder and frustum of a cone
a cone and hemisphere
A surahi is a combination of
a sphere and a cylinder
a hemisphere and a cylinder
a cylinder and a cone
two hemispheres
The shape of the gilli used in a gilli-danda game is a combination of
a cone and a cylinder
two cylinders
two cones and a cylinder
two cylinders and a cone
A plumbline (sahul) is a combination of

a hemisphere and a cone
a cylinder and a cone
a cylinder and frustum of a cone
a cylinder and a sphere
A cone is cut by a plane parallel to its base and the upper part is removed. The part that is left is called

a cone
a sphere
a cylinder
frustum of a cone
During conversion of a solid from one shape to another, the volume of the new shape will ______.
decrease
increase
remain unaltered
remain unaltered
In a right circular cone, the cross-section made by a plane parallel to the base is a
sphere
hemisphere
circle
a semicircle
A solid piece of iron in the form a cuboid of dimensions (49 cm × 33 cm × 24 cm) is moulded into a solid sphere. The radius of the sphere is ______.
19 cm
21 cm
23 cm
25 cm
The radius (in cm) of the largest right circular cone that can be cut out from a cube of edge 4.2 cm is ______.
2.1
4.2
8.4
1.05
Choose the correct answer of the following question:
A metallic solid sphere of radius 9 cm is melted to form a solid cylinder of radius 9 cm. The height of the cylinder is
12 cm
18 cm
36 cm
96 cm
A rectangular sheet of paper 40 cm × 22 cm, is rolled to form a hollow cylinder of height 40 cm. The radius of the cylinder (in cm) is ______.
3.5
7
\[\frac{80}{7}\]
5
Choose the correct answer of the following question:
The number of solid spheres, each of diameter 6 cm, that can be made by melting a solid metal cylinder of height 45 cm and diameter 4 cm, is
2
4
5
5
6
If the surface area of a sphere is 616 cm2, its diameter (in cm) is ______. (Taking π = `22/7`)
7
14
28
56
Choose the correct answer of the following question:
If the radius of a sphere becomes 3 times, then its volume will become
3 times
6 times
9 times
27 times
Choose the correct answer of the following question:
If the height of a bucket in the shape of frustum of a cone is 16 cm and the diameters of its two circular ends are 40 cm and 16 cm, then its slant height is
A sphere of diameter 18 cm is dropped into a cylindrical vessel of diameter 36 cm, partly filled with water. If the sphere is completely submerged, then the water level rises by ______.
3 cm
4 cm
5 cm
6 cm
Choose the correct answer of the following question:
A solid right circular cone is cut into two parts at the middle of its height by a plane parallel to its base. The ratio of the volume of the smaller cone to the whole cone is
1 : 2
1 : 4
1 : 6
1 : 8
Choose the correct answer of the following question:
The radii of the circular ends of a bucket of height 40 cm are 24 cm and 15 cm. The slant height (in cm) of the bucket is
41
43
49
51
Choose the correct answer of the following question:
A solid is hemispherical at the bottom and conical (of same radius) above it. If the surface areas of the two parts are equal, then the ratio of its radius and the slant height of the conical part is
1 : 2
2 : 1
1 : 4
4 : 1
Choose the correct answer of the following question:
If the radius of the base of a right circular cylinder is halved, keeping the height the same, then the ratio of the volume of the cylinder thus obtained to the volume of original cylinder is
1 : 2
2 : 1
1 : 4
4 : 1
A cubical ice-cream brick of edge 22 cm is to be distributed among some children by filling ice-cream cones of radius 2 cm and height 7 cm up to the brim. How many children will get the ice-cream cones?
163
263
363
463
A mason constructs a wall of dimensions (270 cm × 300 cm × 350 cm) with bricks, each of size (22.5 cm × 11.25 cm × 8.75 cm) and it is assumed that `1/8` space is covered by the mortar. Number of bricks used to construct the wall is ______.
11000
11100
11200
11300
Twelve solid spheres of the same size are made by melting a solid metallic cylinder of base diameter 2 cm and height 16 cm. The diameter of each sphere is ______.
2 cm
3 cm
4 cm
6 cm
The diameters of two circular ends of a bucket are 44 cm and 24 cm, and the height of the bucket is 35 cm. The capacity of the bucket is
31.7 liters
32.7 liters
33.7 litres
34.7 litres
The slant height of a bucket is 45 cm and the radii of its top and bottom are 28 cm and 7 cm, respectively. The curved surface area of the bucket is
4953 cm2
4952 cm2
4951 cm2
4950 cm2
The volumes of two spheres are in the ratio 64 : 27. The ratio of their surface areas is ______.
9 : 16
16 : 9
3 : 4
4 : 3
A hollow cube of internal edge 22 cm is filled with spherical marbles of diameter 0.5 cm and `1/8` space of the cube remains unfilled. Number of marbles required is
142296
142396
142396
142596
A metallic spherical shell of internal and external diameters 4 cm and 8 cm, respectively, is melted and recast in the form of a cone of base diameter 8 cm. The height of the cone is ______.
12 cm
14 cm
15 cm
8 cm
A medicine capsule is in the shape of a cylinder of diameter 0.5 cm with a hemisphere tucked at each end. The length of the entire capsule is 2 cm. The capacity of the capsule is
The length of the longest pole that can be kept in a room (12 m × 9 m ×8 m) is
29 m
21 m
19 m
17 m
The length of the longest pole that can be kept in a room (12 m × 9 m ×8 m) is
144 cm2
216 cm2
180 cm2
108 cm2
The volume of a cube is 2744 cm2. Its surface area is
196 cm2
1176 cm2
784 cm2
588 cm2
The total surface area of a cube is 864 cm2. Its volume is
3456 cm3
432 cm3
1728 cm3
3456 cm3
How many bricks, each measuring (25 cm × 11.25 cm × 6 cm), will be required to construct a wall (8 m × 6 m × 22.5 cm)?
8000
6400
4800
7200
The area of the base of a rectangular tank is 6500 cm2 and the volume of water contained in it is 2.6 m3. The depth of water in the tank is
3.5 m
4 m
5 m
8 m
The volume of a wall, 5 times as high as it is broad and 8 times as long as it is high, is 128 m3. The breadth of the wall is
30 cm
40 cm
22.5 cm
25 cm
If the areas of three adjacent faces of a cuboid are x, y and z, respectively, the volume of the cuboid is ______.
xyz
2xyz
`sqrt("xyz")`
`root(3)("xyz")`
The sum of length, breadth and height of a cuboid is 19 cm and its diagonal is `5sqrt(5)` cm. Its surface area is
361 cm2
125 cm2
236 cm2
486 cm2
If each edge of a cube is increased by 50%, the percentage increase in the surface area is
50%
75%
100%
125%
How many bags of grain can be stored in a cuboidal granary (8 m × 6 m × 3 m), if each bag occupies a space of 0.64 m3?
8256
90
212
225
A cube of side 6 cm is cut into a number of cubes, each of side 2 cm. The number of cubes formed is
6
9
12
27
Rainfall in an area is 5 cm. The volume of the water that falls on 2 hectares of land is
100 m3
10 m3
1000 m3
10000 m3
The volumes of two cubes are in the ratio 1 : 27. The ratio of their surface area is
1 : 3
1 : 8
1 : 9
1 : 18
The diameter of the base of a cylinder is 4 cm and its height is 14 cm. The volume of the cylinder is
176 cm3
196 cm3
276 cm3
352 cm3
The diameter of a cylinder is 28 cm and its height is 20 cm. The total surface area of the cylinder is
2993 cm2
2992 cm2
2292 cm2
2229 cm2
πThe height of a cylinder is 14 cm and its curved surface area is 264 cm2. The volume of the cylinder is
308 cm3
396 cm3
1232 cm3
1848 cm3
The curved surface are of a cylinder is 1760 cm2 and its base radius is 14 cm. The height of the cylinder is
10 cm
15 cm
20 cm
40 cm
The ratio of the total surface area to the lateral surface area of a cylinder with base radius 80 cm and height 20 cm is
2 : 1
3 : 1
4 : 1
5 : 1
The curved surface area of a cylindrical pillar is 264 m2 and its volume is 924 m3. The height of the pillar is
4 m
5 m
6 m
7 m
The ratio between the radius of the base and the height of a cylinder is 2 : 3. If its volume is 1617 cm3, the total surface area of the cylinder is
308 cm2
462 cm2
540 cm2
770 cm2
The radii of two cylinders are in the ratio 2 : 3 and their heights are in the ratio 5 : 3. The ratio of their volumes is ______.
27 : 20
20 : 27
4 : 9
9 : 4
The heights of two circular cylinders of equal volume are in the ratio 1 : 2. The ratio of their radii is
`1 : sqrt(2)`
`sqrt(2) : 1`
1 : 2
1 : 4
The radius of the base of a cone is 5 cm and its height is 12 cm. Its curved surface area is
60π cm2
65π cm2
30π cm2
None of these
The diameter of the base of a cone is 42 cm and its volume is 12936 cm3. Its height is
28 cm
21 cm
35 cm
14 cm
The area of the base of a right circular cone is 154 cm2 and its height is 14 cm. Its curved surface area is
154`sqrt(5) "cm"^2`
`154 sqrt(7) "cm"^2`
`77sqrt(7) "cm"^2`
`77sqrt(5) "cm"^2`
On increasing the radii of the base and the height of a cone by 20%, its volume will increase by
20%
40%
60%
72.8%
The radii of the base of a cylinder and a cone are in the ratio 3 : 4. If their heights are in the ratio 2 : 3, the ratio between their volumes is
9 : 8
3 : 4
8 : 9
4 : 3
A metallic cylinder of radius 8 cm and height 2 cm is melted and converted into a right circular cone of height 6 cm. The radius of the base of this cone is
4 cm
5 cm
6 cm
8 cm
The height of a conical tent is 14 m and its floor area is 346.5 m2. How much canvas, 1.1 wide, will be required for it?
490 m
525 m
665 m
860 m
The diameter of a sphere is 14 cm. Its volume is
1428 cm3
1439 cm3
`1437 1/3 "cm"^3`
1440 cm3
The ratio between the volume of two spheres is 8 : 27. What is the ratio between their surface areas?
2 : 3
4 : 5
5 : 6
4 : 9
A hollow metallic sphere with external diameter 8 cm and internal diameter 4 cm is melted and moulded into a cone of base radius 8 cm. The height of the cone is
12 cm
14 cm
15 cm
18 cm
3 . 5 cm
A metallic cone of base radius 2.1 cm and height 8.4 cm is melted and moulded into a sphere. The radius of the sphere is
2.1 cm
1.05 cm
1.5 cm
2 cm
The surface area of a sphere is 154 cm2. The volume of the sphere is
`"179"2/3 "cm"^3`
`"359"1/3"cm"^3`
`"1437"1/3"cm"^3`
None of these
The total surface area of a hemisphere of radius 7 cm is
(588π) cm2
(392π) cm2
(147π) cm2
(98π) cm2
The circular ends of a bucket are of radii 35 cm and 14 cm and the height of the bucket is 40 cm. Its volume is
60060 cm3
80080 cm3
70040 cm3
80160 cm3
If the radii of the ends of a bucket are 5 cm and 15 cm and it is 24 cm high, then its surface area is
1815.3 cm2
1711.3 cm2
2025.3cm2
2360 cm2
A circus tent is cylindrical to a height of 4 m and conical above it. If its diameter is 105 m and its slant height is 40 m, the total area of canvas required is
1760 m2
2640 m2
3960 m2
7920 m2
Match the following columns:
| Column I | Column II |
| (a) A solid metallic sphere of radius 8 cm is melted and the material is used to make solid right cones with height 4 cm and base radius of 8 cm. How many cones are formed? | (p) 18 |
| (b) A 20-m-deep well with diameter 14 m is dug up and the earth from digging is evenly spread out to form a platform 44 m by 14 m. The height of the platform is ...........m. |
(q) 8 |
| (c) A sphere of radius 6 cm is melted and recast in the shape of a cylinder of radius 4 cm. Then, the height of the cylinder is ......... cm. |
(r) 16 : 9 |
| (d) The volumes of two spheres are in the ratio 64 : 27. The ratio of their surface areas is ....... . |
(s) 5 |
Match the following columns:
| Column I | Column II |
| (a) The radii of the circular ends of a bucket, in the form of the frustum of a cone of height 30 cm, are 20 cm and 10 cm respectively. The capacity of the bucket is ........cm3. |
(p) 2418π |
| (b) The radii of the circular ends of a conical bucket of height 15 cm are 20 and 12 cm respectively. The slant height of the bucket is ........ cm. |
(q) 22000 |
| (c) The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm and its slant height is 10 cm. The total surface area of the bucket is .........cm2. |
(r) 12 |
| (d) Three solid metallic spheres of radii 3 cm, 4 cm and 5 cm are melted to form a single solid sphere. The diameter of the resulting sphere is ........ cm. |
(s) 17 |
Assertion (A)
If the radii of the circular ends of a bucket 24 cm high are 15 cm and 5 cm, respectively, then the surface area of the bucket is 545π cm2.
- Reason(R)
If the radii of the circular ends of the frustum of a cone are R and r, respectively, and its height is h, then its surface area is - Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
Assertion (A)
The outer surface of a hemisphere of radius 7 cm is to be painted. The total cost of painting at Rs 5 per cm2 is Rs 2300.
Reason (R)
The total surface area of a hemisphere is 3π r2.
- Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).
- Both Assertion (A) and Reason (R) are true but Reason (R) is not a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
Assertion (A)
If the volumes of two spheres are in the ratio 27 : 8, then their surface areas are in the ratio 3 : 2.
Reason (R)
Volume of a sphere `=4/3pi"R"^3`
Surface area of a sphere = 4πR2
Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).- Both Assertion (A) and Reason (R) are true but Reason (R) is not a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
Assertion (A)
The curved surface area of a cone of base radius 3 cm and height 4 cm is 15π cm2.\
Reason (R)
Volume of a cone = πr2h
- Both Assertion (A) and Reason (R) are true and Reason (R) is a correct explanation of Assertion (A).
- Both Assertion (A) and Reason (R) are true but Reason (R) is not a correct explanation of Assertion (A).
- Assertion (A) is true and Reason (R) is false.
- Assertion (A) is false and Reason (R) is true.
RS Aggarwal solutions for Mathematics [English] Class 10 19 Volume and Surface Area of Solids Formative Assessment [Pages 937 - 938]
Find the number of solid spheres, each of diameter 6 cm, that could be moulded to form a solid metallic cylinder of height 45 cm and diameter 4 cm.
Two right circular cylinders of equal volumes have their heights in the ratio 1 : 2. What is the ratio of their radii?
A circus tent is cylindrical to a height of 4 m and conical above it. If its diameter is 105 m and its slant height is 40 m, then find the total area of the canvas required.
The radii of the top and bottom of a bucket of slant height 45 cm are 28 cm and 7 cm, respectively. Find the curved surface area of the bucket.
A solid metal cone with base radius 12 cm and height 24 cm is melted to form solid spherical balls of diameter 6 cm each. Find the number of balls formed.
A hemispherical bowl of internal diameter 30 cm is full of a liquid. This liquid is poured into cylindrical bottles of diameter 5 cm and height 6 cm each. How many bottles are required?
A solid metallic sphere of diameter 21 cm is melted and recast into small cones of diameter 3.5 cm and height 3 cm each. Find the number of cones so formed.
The diameter of a sphere is 42 cm. It is melted and drawn into a cylindrical wire of diameter 2.8 cm. Find the length of the wire.
A drinking glass is in the shape of the frustum of a cone of height 21 cm with 6 cm and 4 cm as the diameters of its two circular ends. Find the capacity of the glass.
Two cubes, each of volume 64 cm3, are joined end to end. Find the total surface area of the resulting cuboid.
The radius of the base and the height of a solid right circular cylinder are in the ratio 2 : 3 and its volume is 1617 cm3. Find the total surface area of the cylinder.
A toy is in the form of a cone mounted on a hemisphere of common base radius 7 cm. The total height of the toy is 31 cm. Find the total surface area of the toy.
A hemispherical bowl of internal radius 9 cm is full of water. This water is to be filled in cylindrical bottles of diameter 3 cm and height 4 cm. Find the number of bottles needed in which the water can be filled.
The surface areas of a sphere and a cube are equal. Find the ratio of their volumes.
The slant height of the frustum of a cone is 4 cm and the perimeters (i.e. circumferences) of its circular ends are 18 cm and 6 cm. Find the curved surface area of the frustum.
A solid is composed of a cylinder with hemispherical ends. If the whole length of the solid is 104 cm and the radius of each hemispherical end is 7 cm, find the surface area of the solid.
From a solid cylinder whose height is 15 cm and diameter 16 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid. (Use π = 3.14)
A solid rectangular block of dimensions 4.4 m, 2.6 m and 1 m is cast into a hollow cylindrical pipe of internal radius 30 cm and thickness 5 cm. Find the length of the pipe.
An open metal bucket is in the shape of a frustum of a cone, mounted on a hollow cylindrical base made of the same metallic sheet. The diameters of the two circular ends of the bucket are 45 cm and 25 cm, the total vertical height of the bucket is 40 cm and that of the cylindrical base is 6 cm. Find the area of the metallic sheet used to make the bucket. Also, find the volume of water the bucket can hold, in litres.
A farmer connects a pipe of internal diameter 20 cm from a canal into a cylindrical tank which is 10 m in diameter and 2 m deep. If the water flows through the pipe at the rate of 4 km/hr, then in how much time will the tank be filled completely?
Solutions for 19: Volume and Surface Area of Solids
![RS Aggarwal solutions for Mathematics [English] Class 10 chapter 19 - Volume and Surface Area of Solids RS Aggarwal solutions for Mathematics [English] Class 10 chapter 19 - Volume and Surface Area of Solids - Shaalaa.com](/images/9789350271810-mathematics-english-class-10_6:362518cae02c41f896f78fdfb22bac39.jpg)
RS Aggarwal solutions for Mathematics [English] Class 10 chapter 19 - Volume and Surface Area of Solids
Shaalaa.com has the CBSE Mathematics Mathematics [English] Class 10 CBSE solutions in a manner that help students grasp basic concepts better and faster. The detailed, step-by-step solutions will help you understand the concepts better and clarify any confusion. RS Aggarwal solutions for Mathematics Mathematics [English] Class 10 CBSE 19 (Volume and Surface Area of Solids) include all questions with answers and detailed explanations. This will clear students' doubts about questions and improve their application skills while preparing for board exams.
Further, we at Shaalaa.com provide such solutions so students can prepare for written exams. RS Aggarwal textbook solutions can be a core help for self-study and provide excellent self-help guidance for students.
Concepts covered in Mathematics [English] Class 10 chapter 19 Volume and Surface Area of Solids are Volume of a Combination of Solids, Frustum of a Cone, Surface Area of a Combination of Solids, Conversion of Solid from One Shape to Another, Concept of Surface Area, Volume, and Capacity, Surface Area and Volume of Different Combination of Solid Figures, Surface Area and Volume of Three Dimensional Figures, Volume of a Combination of Solids, Frustum of a Cone, Surface Area of a Combination of Solids, Conversion of Solid from One Shape to Another, Concept of Surface Area, Volume, and Capacity, Surface Area and Volume of Different Combination of Solid Figures, Surface Area and Volume of Three Dimensional Figures.
Using RS Aggarwal Mathematics [English] Class 10 solutions Volume and Surface Area of Solids exercise by students is an easy way to prepare for the exams, as they involve solutions arranged chapter-wise and also page-wise. The questions involved in RS Aggarwal Solutions are essential questions that can be asked in the final exam. Maximum CBSE Mathematics [English] Class 10 students prefer RS Aggarwal Textbook Solutions to score more in exams.
Get the free view of Chapter 19, Volume and Surface Area of Solids Mathematics [English] Class 10 additional questions for Mathematics Mathematics [English] Class 10 CBSE, and you can use Shaalaa.com to keep it handy for your exam preparation.
