Advertisements
Advertisements
प्रश्न
How many bricks, each measuring (25 cm × 11.25 cm × 6 cm), will be required to construct a wall (8 m × 6 m × 22.5 cm)?
विकल्प
8000
6400
4800
7200
उत्तर
6400
Volume of the wall = (800 xx 600 xx 22.5) cm3
Volume of each brick = (25×11.25×6) cm3
Number of bricks `= "Volume of the wall"/"Volume of each brick "`
`= (800xx600xx22.5)/(25xx11.25xx6)`
= 6400
APPEARS IN
संबंधित प्रश्न
A heap of rice is in the form of a cone of base diameter 24 m and height 3.5 m. Find the volume of the rice. How much is canvas cloth required to just cover the heap?
A copper rod of diameter 1cm and length 8cm is drawn into a wire of length 18m of uniform thickness. Find thickness of wire?
The surface area of a solid metallic sphere is 616 cm2. It is melted and recast into a cone of height 28 cm. Find the diameter of the base of the cone so formed (Use it =`22/7`)
A metallic solid right circular cone is of height 84 cm and the radius of its base is 21 cm. It is melted and recast into a solid sphere. Find the
diameter of the sphere.
In the equilateral Δ ABC of side 14 cm, side BC is the diameter of a semicircle as shown in the figure below. Find the area of the shaded region. (Take π = 22/7 and √3 = 1.732)
If the radius of base of a right circular cylinder is halved, keeping the height same, the ratio of the volume of the reduced cylinder to that of the original cylinder is ______.
Two solid cones A and B are placed in a cylinderical tube as shown in the figure. The ratio of their capacities are 2:1. Find the heights and capacities of cones. Also, find the volume of the remaining portion of the cylinder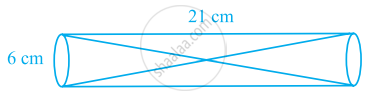
A wall 24 m long, 0.4 m thick and 6 m high is constructed with the bricks each of dimensions 25 cm × 16 cm × 10 cm. If the mortar occupies `1/10` th of the volume of the wall, then find the number of bricks used in constructing the wall.
How many cubic centimetres of iron is required to construct an open box whose external dimensions are 36 cm, 25 cm and 16.5 cm provided the thickness of the iron is 1.5 cm. If one cubic cm of iron weighs 7.5 g, find the weight of the box.
Find the volume of a solid hemisphere whose total surface area is 462 sq.m.
