Advertisements
Advertisements
प्रश्न
A solid metallic sphere of diameter 21 cm is melted and recast into a number of smaller cones, each of diameter 3.5 cm and height 3 cm. Find the number of cones so formed.
उत्तर
Diameter of sphere = 21 cm
Radius of sphere =`21/2` cm
Volume of sphere `= 4/3 pir^3 = (4xx21xx21xx21xx21pi)/(3xx2xx2xx2)= (21xx21xx21pi)/(3xx2) "cm"^3`
Diameter of the cone = 3.5 cm
Radius of the cone `= 3.5/2 = 7/4 "cm"`
Height = 3 cm
Volume of each cone` = 1/3pir^2h xx 1/3pixx3xx(7/4)^2 = (7/4)^2 pi "cm"^3 `
`"Total number of cones"= "Volume of sphere"/"Volume of a cone" = ((21xx21xx21pi)/(3xx2))/((7/4)^2pi) = (21xx21xx21xxpixx4xx4)/3xx2xxpixx)`
APPEARS IN
संबंधित प्रश्न
504 cones, each of diameter 3.5 cm and height 3 cm, are melted and recast into a metallic sphere. Find the diameter of the sphere and hence find its surface area.
[Use π=22/7]
The largest possible sphere is carved out of a wooden solid cube of side 7 em. Find the volume of the wood left. (Use\[\pi = \frac{22}{7}\]).
From a solid right circular cylinder of height 2.4 cm and radius 0.7 cm, a right circular cone of same height and same radius is cut out. Find the total surface area of the remaining solid.
The radii of the circular bases of a frustum of a right circular cone are 12 cm and 3 cm and the height is 12 cm. Find the total surface area and the volume of the frustum.
The inner and outer radii of a hollow cylinder are 15 cm and 20 cm, respectively. The cylinder is melted and recast into a solid cylinder of the same height. Find the radius of the base of new cylinder.
Water is flowing through a cylindrical pipe of internal diameter 2 cm, into a cylindrical tank of base radius 40 cm, at the rate of 0.4 m per second. Determine the rise in level of water in the tank in half an hour.
Five identical cubes, each of edge 5 cm, are placed adjacent to each other. Find the volume of the resulting cuboid.
A cone of height 24 cm and radius of base 6 cm is made up of modelling clay. A child reshapes it in the form of a sphere. Find the radius of the sphere and hence find the surface area of this sphere.
A tent is in the shape of a cylinder surmounted by a conical top. If the height and radius of the cylindrical part are 3 m and 14 m respectively, and the total height of the tent is 13.5 m, find the area of the canvas required for making the tent, keeping a provision of 26 m2 of canvas for stitching and wastage. Also, find the cost of the canvas to be purchased at the rate of ₹ 500 per m2.
Tamper-proof tetra-packed milk guarantees both freshness and security. This milk ensures uncompromised quality, preserving the nutritional values within and making it a reliable choice for health-conscious individuals.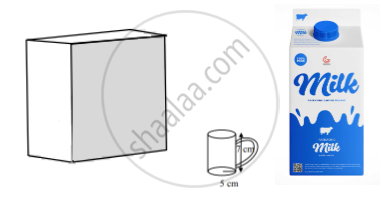
500 ml milk is packed in a cuboidal container of dimensions 15 cm × 8 cm × 5 cm. These milk packets are then packed in cuboidal cartons of dimensions 30 cm × 32 cm × 15 cm.
Based on the above-given information, answer the following questions:
i. Find the volume of the cuboidal carton. (1)
ii. a. Find the total surface area of the milk packet. (2)
OR
b. How many milk packets can be filled in a carton? (2)
iii. How much milk can the cup (as shown in the figure) hold? (1)
