Advertisements
Advertisements
प्रश्न
A solid metallic right circular cone 20 cm high and whose vertical angle is 60°, is cut into two parts at the middle of its height by a plane parallel to its base. If the frustum so obtained be drawn into a wire of diameter `1/12` cm, then find the length of the wire.
उत्तर
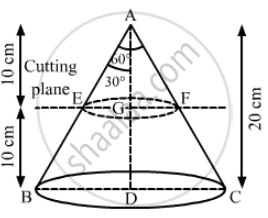
We have,
Height of the metallic cone, H = 20 cm,
Height of the frustum, `"h" = 20/2 = 10 "cm"` and
Radius of the wire `= 1/24 "cm"`
Let the length of the wire be l, EG = r and BD = R
In ΔAEG,
`"tan"30° = "EG"/"AG"`
`rArr 1/sqrt(3) = r/("H"-"h")`
`rArr 1/sqrt(3)="r"/(20-10)`
`rArr r = 10/sqrt(3) "cm"`
Also, in Δ ABD,
`tan 30° = "BD"/"AD"`
`rArr 1/sqrt(3) = "R"/"H"`
`rArr 1/sqrt(3) = "R"/20`
`rArr "R" = 20/sqrt(3) "cm"`
Now,
Volume of the wire = Volume of the frustum
`rArr pi(1/24)^2l = 1/3pih (R^2 + r^2 +"Rr")`
`= "l"/576 = 1/3xx10xx[(20/sqrt(3))^2 + (10/sqrt(3))^2 + (20/sqrt(3))(10/sqrt(3))]`
`rArr "l" = 576/3xx10xx[400/3+100/3+200/3]`
`= "l" = 576/3xx10xx700/3`
⇒ l = 448000 cm
∴ l = 4480 m
So, the length of the wire is 4480 m.
APPEARS IN
संबंधित प्रश्न
A cone of height 20 cm and radius of base 5 cm is made up of modeling clay. A child reshapes it in the form of a sphere. Find the diameter of the sphere.
A milk container of height 16 cm is made of metal sheet in the form of a frustum of a cone with radii of its lower and upper ends as 8 cm and 20 cm respectively . Find the cost of milk at the rate of ₹44 per litre which the container can hold.
A circus tent is cylindrical to a height of 3 metres and conical above it. If its diameter is 105 m and the slant height of the conical portion is 53 m, calculate the length of the canvas 5 m wide to make the required tent.
If a cone is cut into two parts by a horizontal plane passing through the mid-point of its axis, the ratio of the volumes of the upper part and the cone is
A right triangle with sides 3 cm, 4 cm and 5 cm is rotated about the side of 3 cm to form a cone. The volume of the cone so formed is
A solid metal cone with base radius of 12 cm and height 24 cm is melted to form solid spherical balls of diameter 6 cm each. Find the number of balls thus formed.
A solid metallic sphere of diameter 28 cm is melted and recast into a number of smaller cones, each of diameter `"4"2/3` cm and height 3 cm. Find the number of cones so formed.
A cylindrical pencil sharpened at one edge is combination of ______.
A solid formed on revolving a right-angled triangle about its height is ______.
Find the curved surface area of a right circular cone whose height is 15 cm and base radius is 8 cm. tan β = `3/4`.
