Advertisements
Advertisements
प्रश्न
A cone of height 20 cm and radius of base 5 cm is made up of modeling clay. A child reshapes it in the form of a sphere. Find the diameter of the sphere.
उत्तर
Let the radius of the sphere be r.
Radius of the cone = 5 cm
Height of the cone = 20 cm
Since the cone is reshaped to form a sphere, the volumes of the sphere and the cone are equal.
`∴ 4/3pir^3=1/3pi(5cm)^2xx(20 cm)` `(∵ \text{Text of cone =}1/3 piR^2H)`
`rArr r^3=125 cm^3`
`rArr r^3=(5 cm)^3`
`rArr r^3=5 cm`
Therefore, diameter of the sphere = 2r = 2 × 5 cm = 10 cm
APPEARS IN
संबंधित प्रश्न
An oil funnel of tin sheet consists of a cylindrical portion 10 cm long attached to a frustum of a cone. If the total height be 22 cm, the diameter of the cylindrical portion 8 cm and the diameter of the top of the funnel 18 cm, find the area of the tin required.(Use π = 22/7).
The radii of the base of a cylinder and a cone are in the ratio 3 : 4 and their heights are in the ratio 2 : 3. What is the ratio of their volumes?
The material of a cone is converted into the shape of a cylinder of equal radius. If height of the cylinder is 5 cm, then height of the cone is
The radii of the circular ends of a frustum are 6 cm and 14 cm. If its slant height is 10 cm, then its vertical height is
A spherical cannon ball, 28 cm in diameter, is melted and recast into a right circular conical mould with base diameter of 35 cm. Find the height of the cone.
The radii of the circular ends of a solid frustum of a cone are 33 cm and 27 cm and its slant height is 10 cm. Find its total surface area. [Use π = 3.14.]
A tent is made in the form of a frustum of a cone surmounted by another cone. The diameters of the base and the top of the frustum are 20 m and 6 m, respectively, and the height is 24 m. If the height of the tent is 28 m and the radius of the conical part is equal to the radius of the top of the frustum, find the quantity of canvas required.
The radii of the circular ends of a frustum of height 6 cm are 14 cm and 6 cm, respectively. Find the slant height of the frustum.
A drinking glass is in the shape of the frustum of a cone of height 21 cm with 6 cm and 4 cm as the diameters of its two circular ends. Find the capacity of the glass.
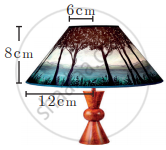
The frustum shaped outer portion of the table lamp has to be painted including the top part. Find the total cost of painting the lamp if the cost of painting 1 sq.cm is ₹ 2.