Advertisements
Advertisements
प्रश्न
The slant height of a conical mountain is 2.5 km and the area of its base is 1.54 km2. Find the height of the mountain.
उत्तर
Let r, h and l be the base radius, the height and the slant height of the conical mountain, respectively.
As, the area of the base = 1.54 Km2
`rArr pir^2 = 1.54`
`rArr 22/7xx r^2 = 1.54`
`rArr r^2 = (1.54xx7)/22`
`rArr r2 = 0.49`
`rArr r = sqrt(0.49)`
`rArr = 0.7 "Km"`
Now ,
`h = sqrt(l^2 - r^2)`
`= sqrt(2.5^2 - 0.7^2)`
`=sqrt(6.25-0.49)`
`= sqrt(5.76)`
`=2.4 "Km"`
So, the height of the mountain is 2.4 km.
Now, the volume of the solid cylinder = πr2 h
`= 22/7 xx 7xx 7xx30`
= 4620 m3
APPEARS IN
संबंधित प्रश्न
A vessel is a hollow cylinder fitted with a hemispherical bottom of the same base. The depth of the cylinder is `14/3` m and the diameter of hemisphere is 3.5 m. Calculate the volume and the internal surface area of the solid.
Radius of a sphere is 14 cm. Find the surface area of the sphere.
A cylindrical tank full of water is emptied by a pipe at the rate of 225 litres per minute. How much time will it take to empty half the tank, if the diameter of its base is 3 m and its height is 3.5 m? [Use \[\pi = \frac{22}{7}\]]
A conical vessel whose internal radius is 10 cm and height 48 cm is full of water. Find the volume of water. If this water is poured into a cylindrical vessel with internal radius 20 cm, find the height to which the water level rises in it.
A cylindrical pencil sharpened at one end is a combination of
Choose the correct answer of the following question:
A solid is hemispherical at the bottom and conical (of same radius) above it. If the surface areas of the two parts are equal, then the ratio of its radius and the slant height of the conical part is
The curved surface are of a cylinder is 1760 cm2 and its base radius is 14 cm. The height of the cylinder is
The capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom as shown in the figure is `(pir^2)/3 [3h - 2r]`.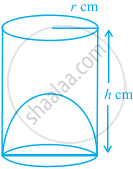
The surface areas of the six faces of a rectangular solid are 16, 16, 32, 32, 72 and 72 square centimetres. The volume of the solid, in cubic centimetres, is ______.
A bicycle wheel makes 500 revolutions in moving 1 km. Find the diameter of the wheel.
