Advertisements
Advertisements
प्रश्न
The capacity of a cylindrical vessel with a hemispherical portion raised upward at the bottom as shown in the figure is `(pir^2)/3 [3h - 2r]`.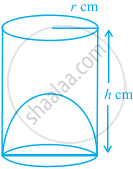
विकल्प
True
False
उत्तर
This statement is True.
Explanation:
We know that,
Capacity of cylindrical vessel = πr2h cm3
And capacity of hemisphere = `2/3 pi"r"^3 "cm"`
From the figure,
Capacity of the cylindrical vessel
= `pi"r"^2"h" - 2/3 pi"r"^3`
= `1/3 pi"r"^2 [3"h" - 2"r"]`
APPEARS IN
संबंधित प्रश्न
Due to heavy floods in a state, thousands were rendered homeless. 50 schools collectively offered to the state government to provide place and the canvas for 1500 tents to be fixed by the governments and decided to share the whole expenditure equally. The lower part of each tent is cylindrical of base radius 2.8 cm and height 3.5 m, with conical upper part of same base radius but of height 2.1 m. If the canvas used to make the tents costs Rs. 120 per sq. m, find the amount shared by each school to set up the tents. What value is generated by the above problem? (use `pi =22/7`)
A bucket is in the form of a frustum of a cone with a capacity of 12308.8 cm3 of water.The radii of the top and bottom circular ends are 20 cm and 12 cm respectively. Find the height of the bucket and the area of the metal sheet used in its making. (Use 𝜋 = 3.14).
Find the total surface area of a cylinder if the radius of its base is 5 cm and height is 40 cm.
A canal is 300 cm wide and 120 cm deep. The water in the canal is flowing with a speed of 20 km/hr. How much area will it irrigate in 20 minutes if 8 cm of standing water is desired ?
Find the mass of a 3.5 m long lead pipe, if the external diameter of the pipe is 2.4 cm, thickness of the metal is 2 mm and the mass of 1 cm3 of lead is 11.4 grams.
Water in a canal, 6 m wide and 1.5 m deep, is flowing at a speed of 4 km/hr. How much area will it irrigate in 10 minutes if 8 cm of standing water is needed for irrigation?
A solid cone of base radius 10 cm is cut into two parts through the midpoint of its height, by a plane parallel to its base. Find the ratio of the volumes of the two parts of the cone.
A medicine capsule is in the shape of a cylinder of diameter 0.5 cm with a hemisphere tucked at each end. The length of the entire capsule is 2 cm. The capacity of the capsule is
The diameter of the base of a cylinder is 4 cm and its height is 14 cm. The volume of the cylinder is
A factory manufactures 120000 pencils daily. The pencils are cylindrical in shape each of length 25 cm and circumference of base as 1.5 cm. Determine the cost of colouring the curved surfaces of the pencils manufactured in one day at Rs 0.05 per dm2.
