Advertisements
Advertisements
प्रश्न
A particle is rotated in a vertical circle by connecting it to a string of length l and keeping the other end of the string fixed. The minimum speed of the particle when the string is horizontal for which the particle will complete the circle is
विकल्प
- \[\sqrt{gl}\]
- \[\sqrt{2gl}\]
- \[\sqrt{3gl}\]
- \[\sqrt{5gl}\]
उत्तर
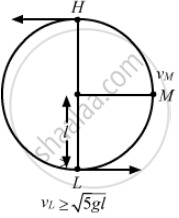
For a complete circle, the minimum velocity at L must be \[v_L = \sqrt{5gl}\] .
Total energy at M = total energy at L
\[ \Rightarrow \frac{1}{2}m {v_M}^2 = \frac{1}{2}m {v_L}^2 - mgl\]
\[\text{ Using } v_L \geq \sqrt{5gl}, \text{ we have} : \]
\[\frac{1}{2}m {v_M}^2 \geq \frac{1}{2}m(5gl) - mgl\]
\[ \therefore v_M = \sqrt{3gl}\]
APPEARS IN
संबंधित प्रश्न
A heavy stone is thrown from a cliff of height h with a speed v. The stoen will hit the ground with maximum speed if it is thrown
A heavy stone is thrown in from a cliff of height h in a given direction. The speed with which it hits the ground
(a) must depend on the speed of projection
(b) must be larger than the speed of projection
(c) must be independent of the speed of projection
(d) may be smaller than the speed of projection.
You lift a suitcase from the floor and keep it on a table. The work done by you on the suitcase does not depend on
(a) the path taken by the suitcase
(b) the time taken by you in doing so
(c) the weight of the suitcase
(d) your weight
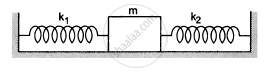
A block of mass m is attached to two unstretched springs of spring constants k1 and k2 as shown in the following figure. The block is displaced towards the right through a distance x and is released. Find the speed of the block as it passes through the mean position shown.
A small heavy block is attached to the lower end of a light rod of length l which can be rotated about its clamped upper end. What minimum horizontal velocity should the block be given so that it moves in a complete vertical circle?
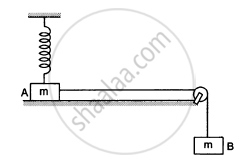
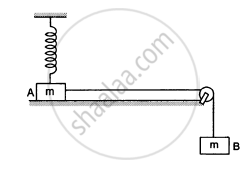
In the following figure shows two blocks A and B, each of mass of 320 g connected by a light string passing over a smooth light pulley. The horizontal surface on which the block Acan slide is smooth. Block A is attached to a spring of spring constant 40 N/m whose other end is fixed to a support 40 cm above the horizontal surface. Initially, the spring is vertical and unstretched when the system is released to move. Find the velocity of the block A at the instant it breaks off the surface below it. Take g = 10 m/s2.
A spring of negligible mass and force constant 5 Nm–1 is compressed by a distance x = 5 cm. A block of mass 200 g is free to leave the end of the spring. If the system is released, what will be the speed of the block when it leaves the spring?
A particle is released from height S from the surface of the Earth. At a certain height, its kinetic energy is three times its potential energy. The height from the surface of the earth and the speed of the particle at that instant are respectively ______.
A particle is released from height S from the surface of the Earth. At a certain height, its kinetic energy is three times its potential energy. The height from the surface of the earth and the speed of the particle at that instant are respectively ______
Which of the diagrams shown in figure represents variation of total mechanical energy of a pendulum oscillating in air as function of time?
A mass of 5 kg is moving along a circular path of radius 1 m. If the mass moves with 300 revolutions per minute, its kinetic energy would be ______.
Why is electrical power required at all when the elevator is descending? Why should there be a limit on the number of passengers in this case?
A body falls towards earth in air. Will its total mechanical energy be conserved during the fall? Justify.
A bob of mass m suspended by a light string of length L is whirled into a vertical circle as shown in figure. What will be the trajectory of the particle if the string is cut at
- Point B?
- Point C?
- Point X?

A baloon filled with helium rises against gravity increasing its potential energy. The speed of the baloon also increases as it rises. How do you reconcile this with the law of conservation of mechanical energy? You can neglect viscous drag of air and assume that density of air is constant.
A single conservative force acts on a body of mass 1 kg that moves along the x-axis. The potential energy U(x) is given by U (x) = 20 + (x - 2)2, where x is in meters. At x = 5.0 m the particle has a kinetic energy of 20 J, then the maximum kinetic energy of body is ______ J.
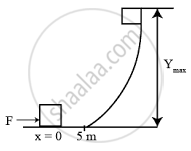
A force shown in the F-x graph is applied to a 5 kg cart, which then coasts up a ramp as shown. The maximum height, ymax is ______ m, at which the cart can reach.
(g = 10 m/s2)