Advertisements
Advertisements
प्रश्न
A pin of length 2.00 cm is placed perpendicular to the principal axis of a converging lens. An inverted image of size 1.00 cm is formed at a distance of 40.0 cm from the pin. Find the focal length of the lens and its distance from the pin.
Advertisements
उत्तर
Given,
Height of the object, (h0) = 2 cm,
Height of the image, (h1) = 1 cm
distance between image and object, ( \[-\] u + v) = 40 cm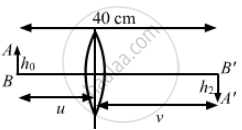 
We know that,
Magnification is given by:
\[m = \frac{h_i}{h_0} = \frac{v}{u}, \frac{1}{2} = \frac{v}{- u}\]
u = −2v
Using lens formula,
\[\frac{1}{v} - \frac{1}{u} = \frac{1}{f}\]
\[ \Rightarrow \frac{1}{v} - \frac{1}{- 2v} = \frac{1}{f}\]
\[ \Rightarrow \frac{1}{v} + \frac{1}{2v} = \frac{1}{f}\]
\[ \Rightarrow f = \frac{2v}{3}\]
As per the question,
= −u + v = 40 cm,
= −(−2v) + v = 40 cm,
= 3v = 40 cm
\[v = 13 . 3 = \frac{40}{3} cm, u = \frac{80}{3}\]
∴ f = 8.9 cm
Hence, the required focal length is 8.9 cm and object distance is 26.66 cm.
APPEARS IN
संबंधित प्रश्न
Define the power of a lens.
What is the focal length of a convex lens of focal length 30 cm in contact with a concave lens of focal length 20 cm? Is the system a converging or a diverging lens? Ignore thickness of the lenses.
Which type of lens has a positive power?
The power of a lens is, −2 D. What is its focal length?
A diverging lens has a focal length of 0.10 m. The power of this lens will be:
Define the term focal length of a lens.
Name the type of lens whose power is positive.
The image of an object formed by a lens is real, inverted and of the same size as the object. If the image is at a distance of 40 cm from the lens, what is the nature and power of the lens ? Draw ray diagram to justify your answer.
A thin converging lens is formed with one surface convex and the other plane. Does the position of image depend on whether the convex surface or the plane surface faces the object?
Consider three converging lenses L1, L2 and L3 having identical geometrical construction. The index of refraction of L1 and L2 are \[\mu_1 \text{ and } \mu_2\] respectively. The upper half of the lens L3 has a refractive index \[\mu_1\] and the lower half has \[\mu_2\] following figure . A point object O is imaged at O1 by the lens L1 and at O2 by the lens L2placed in same position. If L3 is placed at the same place,
(a) there will be an image at O1
(b) there will be an image at O2.
(c) the only image will form somewhere between O1 and O2
(d) the only image will form away from O2.
A screen is placed a distance 40 cm away from an illuminated object. A converging lens is placed between the source and the screen and its is attempted to form the image of the source on the screen. If no position could be found, the focal length of the lens
Surabhi from std. X uses spectacle. The power of the lenses in her spectacle is 0.5 D.
Answer the following questions from the given information:
- Identify the type of lenses used in her spectacle.
- Identify the defect of vision Surabhi is suffering from.
- Find the focal length of the lenses used in her spectacle.
How is accommodation produced?
The power of a lens is +2.0 D. Find its focal length and state the kind of lens.
The power of lens depends on the focal length of the lens
Match the following:
| Column - I | Column - II | ||
| 1 | Retina | a | Path way of light |
| 2 | Pupil | b | Far point comes closer |
| 3 | Ciliary muscles | c | near point moves away |
| 4 | Myopia | d | Screen of the eye |
| 5 | Hypermetropia | e | Power of accommodation |
Define power of a lens. What is its unit? One student uses a lens of focal length 50 cm and another of –50 cm. What is the nature of the lens and its power used by each of them?
The focal length of a concave lens is 20 cm. The focal length of a convex lens is 25 cm. These two are placed in contact with each other. What is the power of the combination? Is it diverging, converging or undeviating in nature?
A point object is placed at O in front of a glass sphere as shown in figure.
Show the formation of the image by the sphere.
The deviation of the incident light rays produced by a lens on refraction through it is a measure of its:
When a lens is placed in a liquid, the total power of the lens is:
