Advertisements
Advertisements
प्रश्न
A ray of monochromatic light passes through an equilateral glass prism in such a way that the angle of incidence is equal to the angle of emergence and each of these angles is 3/4 times the angle of the prism. Determine the angle of deviation and the refractive index of the glass prism.
उत्तर
Here the angle of prism A = 60°, the angle of incidence i = angle of emergence e and under this condition angle of deviation is minimum.
∴ i = e = `3/4` A = `3/4 xx 60^circ = 45^circ` and i + e = A + D,
hence Dm = 2i - A = 2 × 45° - 60° = 30°
The refractive index of the glass prism,
n = `sin((A + D_m)/2)/(sin(A/2)) = (sin((60^circ + 30^circ)/2))/(sin(60^circ/2))`
= `(sin45^circ)/(sin30^circ) = (1/sqrt2)/(1/2) = sqrt2`
APPEARS IN
संबंधित प्रश्न
A ray of light passing from air through an equilateral glass prism undergoes minimum deviation when the angle of incidence is 3/4 th of the angle of prism. Calculate the speed of light in the prism.
Find the angle of incidence at face AB so that the emergent ray grazes along the face AC.
If a piece of paper is placed at the position of a virtual image of a strong light source, will the paper burn after sufficient time? What happens if the image is real? What happens if the image is real but the source is virtual?
A prism is made of glass of unknown refractive index. A parallel beam of light is incident on the face of the prism. The angle of minimum deviation is measured to be 40°. What is the refractive index of the material of the prism? The refracting angle of the prism is 60°. If the prism is placed in water (refractive index 1.33), predict the new angle of minimum deviation of a parallel beam of light.
The refractive index of a prism whose angle A = 60° is `sqrt2`. Then the angle of minimum deviation δm will be ______.
An isosceles prism of angle 120° has a refractive index 1.44. Two parallel monochromatic rays enter the prism parallel to each other in air as shown. The rays emerge from the opposite faces:
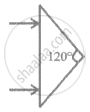
For a glass prism `(µ = sqrt(3))` the angle of minimum deviation is equal to the angle of the prism. Find the angle of the prism.
Two prisms ABC and DBC are arranged as shown in the figure.

The critical angles for the two prisms with respect to air are 41.1° and 45° respectively. Trace the path of the ray through the combination.
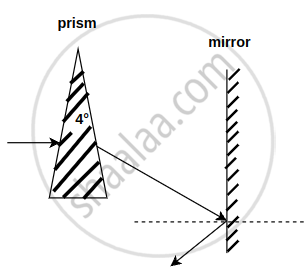
A horizontal ray of light passes through a prism of index 1.50 and apex angle 4° and then strikes a vertical mirror, as shown in the figure (a). Through what angle must the mirror be rotated if after reflection the ray is to be horizontal?
A ray of light is refracted by a glass prism. Obtain an expression for the refractive index of the glass in terms of the angle of prism A and the angle of minimum deviation δm.
