Advertisements
Advertisements
प्रश्न
A right-angled triangle whose sides are 6 cm, 8 cm and 10 cm is revolved about the sides containing the right angle in two ways. Find the difference in volumes of the two solids so formed.
उत्तर
Three sides of a triangle are 6 cm, 8 cm and 10 cm.
Case (i): If the triangle is revolved about the side 6 cm, the cone will be formed with radius 6 cm and height 8 cm.
Volume of the cone = `1/3 pi"r"^2"h"` cu. units
= `1/3 xx pi xx 6 xx 6 xx 8`
= 96π cm3
Case (ii): If the triangle is revolved about the side 8 cm, the cone will be formed with radius 8 cm and height 6 cm.
Volume of the cone = `1/3 xx pi xx 8 xx 8 xx 6`
= 128π cm3
Difference in volume of the two solids
= (128π – 96π) cm3
= 32π cm3
= `32 xx 22/7` cm3
= 100.57 cm3
The difference in the volume of the two solids = 100.57 cm3
APPEARS IN
संबंधित प्रश्न
A cylindrical container with diameter of base 42 cm contains sufficient water to submerge a rectangular solid of iron with dimensions 22 cm × 14 cm × 10.5 cm. Find the rise in the level of the water when the solid is submerged.
The inner radius of a pipe is 2.1 cm. How much water can 12 m of this pipe hold?
A solid metallic sphere of radius 6 cm is melted and made into a solid cylinder of height 32 cm. Find the:
(i) radius of the cylinder
(ii) curved surface area of the cylinder
Take π = 3.1
The total surface area of a solid cylinder is 616 cm2. If the ratio between its curved surface area and total surface area is 1 : 2; find the volume of the cylinder.
The total surface area of a hollow cylinder, which is open from both the sides, is 3575 cm2; area of its base ring is 357.5 cm2 and its height is 14 cm. Find the thickness of the cylinder.
Two right circular solid cylinders have radii in the ratio 3 : 5 and heights in the ratio 2 : 3. Find the ratio between their curved surface areas.
Two right circular solid cylinders have radii in the ratio 3 : 5 and heights in the ratio 2 : 3. Find the ratio between their volumes.
The volume of a cylinder becomes ______ the original volume if its radius becomes half of the original radius.
Two cylinders of same volume have their radii in the ratio 1 : 6, then ratio of their heights is ______.
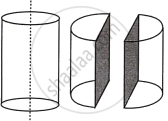
A solid metallic cylinder is cut into two identical halves along its height (as shown in the diagram). The diameter of the cylinder is 7 cm and the height is 10 cm.
Find:
- The total surface area (both the halves).
- The total cost of painting the two halves at the rate of ₹ 30 per cm2 `("Use" π = 22/7)`.