Advertisements
Advertisements
प्रश्न
A Rose garden is in the form of circle of radius 63 m. The gardener wants to fence it at the rate of ₹ 150 per metre. Find the cost of fencing?
उत्तर
Radius of the garden r = 63 m
Circumference of the garden = 2πr units
= `2 xx 22/7 xx 63 "m"`
= 2 × 22 × 9
= 396 m
Cost of fencing 1 meter = ₹ 150
Cost of fencing 396 meter = ₹ 150 × 396 = ₹ 59,400
∴ Cost of fencing the garden = ₹ 59,400
APPEARS IN
संबंधित प्रश्न
A pendulum swings through an angle of 30º and describes an arc 8.8 cm in length. Find the length of the pendulum.
The radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
The wheels of a car are of diameter 80 cm each. How many complete revolutions does each wheel make in 10 minutes when the car is traveling at a speed of 66 km per hour? [Use Π = 22/7]
Shazli took a wire of length 44 cm and bent it into the shape of a circle. Find the radius of that circle. Also find its area. If the same wire is bent into the shape of a square, what will be the length of each of its sides? Which figure encloses more area, the circle or the square? (Take `pi = 22/7`)
The hypotenuse of a right-angled triangle is 65 cm and its base is 60 cm. Find the length of perpendicular and the area of the triangle.
Find the length of the arc of a circle of diameter 42 cm which subtends an angle of 60° at the centre.
The circumferences of two circles are in the ratio 2: 3. What is the ratio between their areas?
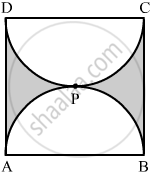
Find the perimeter of the shaded region in the figure, if ABCD is a square of side 14 cm and APB and CPD are semicircles.
A wire when bent in the form of a square encloses an area of 484 cm2. If the same wire is bent into the form of a circle, find the area of the circle.
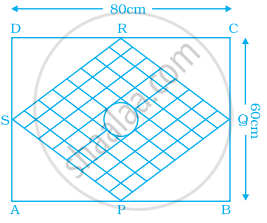
ABCD is a given rectangle with length as 80 cm and breadth as 60 cm. P, Q, R, S are the midpoints of sides AB, BC, CD, DA respectively. A circular rangoli of radius 10 cm is drawn at the centre as shown in the given figure. Find the area of shaded portion.