Advertisements
Advertisements
प्रश्न
A stone is allowed to fall from the top of a tower 100 m high and at the same time another stone is projected vertically upwards from the ground with a velocity of 25 m/s. Calculate when and where the two stones will meet.
Advertisements
उत्तर
Here, h = 100 m
Let the two stones meet after t seconds at point P, which is at a height x above the ground, as shown in the figure.
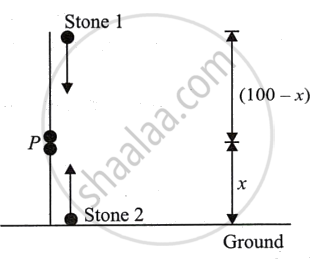
For stone 1,
u = 0, h = (100 - x) m,
a = g = 9.8 m/s2
From s = `ut + 1/2 at^2`
(100 - x) = `0 + 1/2 xx 9.8 t^2`
= 4.9t2 ...(i)
For stone 2,
u = 25 m/s, h = x,
a = - g = - 9.8 m/s2
From s = `ut + 1/2 at^2`
x = `25t + 1/2 (-9.8)t^2`
= 25t - 4.9t2 ...(ii)
Adding equations (i) and (ii)
100 - x + x = 25t
⇒ t = `100/25`
= 4 s
From equation (i),
100 - x = 4.9 × (4)2
100 - x = 78.4
x = 100 - 78.4
x = 21.6 m
APPEARS IN
संबंधित प्रश्न
State whether the following statement is true or false :
A falling stone also attracts the earth.
What is the force of gravity on a body of mass 150 kg lying on the surface of the earth? (Mass of earth = 6 x 1024 kg; Radius of earth = 6.4 x 106 m; G = 6.7 x 10-11 Nm2/kg2)
The values of g at six distances A, B, C, D, E and F from the surface of the earth are found to be 3.08 m/s2, 9.23 m/s2, 0.57 m/s2, 7.34 m/s2, 0.30 m/s2 and 1.49 m/s2, respectively.
(a) Arrange these values of g according to the increasing distances from the surface of the earth (keeping the value of g nearest to the surface of the earth first)
(b) If the value of distance F be 10000 km from the surface of the earth, state whether this distance is deep . inside the earth or high up in the sky. Give reason for your answer.
A ball falls off a table and reaches the ground in 1 s. Assuming g=10 m/s2, calculate its speed on reaching the ground and the height of the table.
Choose one or more correct alternatives.
Which of the forces involved in dragging a heavy object on a smooth, horizontal surface, have the same magnitude?
- The horizontal applied force
- gravitational force
- reaction force in vertical direction
- force of friction
A force acts for 0.1 s on a body of mass 2.0 kg initially at rest. The force is then withdrawn and the body moves with a velocity of 2 m s-1. Find the magnitude of the force.
What force, in newton, your muscles need to apply to hold a mass of 5 kg in your hand? State the assumption.
State whether the below statement is True or False.
Neglecting air resistance, a body falling freely near the earth's surface has a constant acceleration.
The free fall of an object is possible only in ______.
The mass and weight of an object on earth are 5 kg and 49 N respectively. What will be their values on the moon? Assume that the acceleration due to gravity on the moon is 1/6th of that on the earth.
