Advertisements
Advertisements
प्रश्न
A straight horizontal conducting rod of length 0.45 m and mass 60 g is suspended by two vertical wires at its ends. A current of 5.0 A is set up in the rod through the wires.
(a) What magnetic field should be set up normal to the conductor in order that the tension in the wires is zero?
(b) What will be the total tension in the wires if the direction of current is reversed keeping the magnetic field same as before?
(Ignore the mass of the wires) g = 9.8 m s–2.
उत्तर
Length of the rod, l = 0.45 m
Mass suspended by the wires, m = 60 g = 60 × 10−3 kg
Acceleration due to gravity, g = 9.8 m/s2
Current in the rod flowing through the wire, I = 5 A
(a) Magnetic field (B) is equal and opposite to the weight of the wire i.e.,
BIl = mg
∴ B = `"mg"/"Il"`
= `(60 xx 10^-3 xx 9.8)/(5 xx 0.45)`
= 0.26 T
A horizontal magnetic field of 0.26 T normal to the length of the conductor should be set up in order to get zero tension in the wire. The magnetic field should be such that Fleming’s left-hand rule gives an upward magnetic force.
(b) If the direction of the current is reversed, then the force due to the magnetic field and the weight of the wire act in a vertically downward direction.
∴ Total tension in the wire = BIl + mg
= 0.26 × 5 × 0.45 + (60 × 10–3) × 9.8
= 1.176 N
APPEARS IN
संबंधित प्रश्न
Which of the following particles will have minimum frequency of revolution when projected with the same velocity perpendicular to a magnetic field?
A vertical wire carries a current in upward direction. An electron beam sent horizontally towards the wire will be deflected
Two parallel wires carry currents of 20 A and 40 A in opposite directions. Another wire carying a current anti parallel to 20 A is placed midway between the two wires. T he magnetic force on it will be
Consider a non-conducting plate of radius r and mass m that has a charge q distributed uniformly over it. The plate is rotated about its axis with an angular speed ω. Show that the magnetic moment µ and the angular momentum l of the plate are related as `mu = q/(2 m)l`
Figure shows a part of an electric circuit. The wires AB, CD and EF are long and have identical resistance. The separation between the neighbouring wires is 1.0 cm. The wires AE and BF have negligible resistance and the ammeter reads 30 A. Calculate the magnetic force per unit length of AB and CD.

A long, straight wire is fixed horizontally and carries a current of 50.0 A. A second wire having linear mass density 1.0 × 10−4 kg m−1 is placed parallel to and directly above this wire at a separation of 5.0 mm. What current should this second wire carry such that the magnetic repulsion can balance its weight?
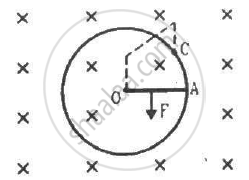
Consider the situation shown in the figure. Suppose the circular loop lies in a vertical plane. The rod has a mass m. The rod and the loop have negligible resistances but the wire connecting O and C has a resistance R. The rod is made to rotate with a uniform angular velocity ω in the clockwise direction by applying a force at the midpoint of OA in a direction perpendicular to it. Find the magnitude of this force when the rod makes an angle θ with the vertical.
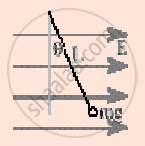
A small object with charge q and weight mg is attached to one end of a string of length ‘L’ attached to a stationary support. The system is placed in a uniform horizontal electric field ‘E’, as shown in the accompanying figure. In the presence of the field, the string makes a constant angle θ with the vertical. The sign and magnitude of q ______.
A straight conductor of length 2m moves at a speed of 20 m/s. When the conductor makes an angle of 30° with the direction of magnetic field of induction of 0.1 wbm2 then induced emf:
A conducting ring of radius 1m kept in a uniform magnetic field B of 0.01 T, rotates uniformly with an angular velocity 100 rad s−1 with its axis of rotation perpendicular to B. The maximum induced emf in it is:
