Advertisements
Advertisements
प्रश्न
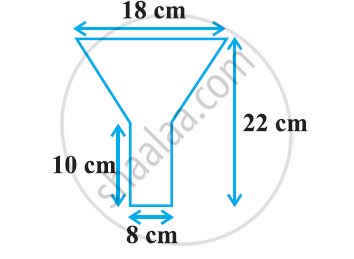
An oil funnel made of tin sheet consists of a 10 cm long cylindrical portion attached to a frustum of a cone. If the total height is 22 cm, diameter of the cylindrical portion is 8 cm and the diameter of the top of the funnel is 18 cm, find the area of the tin sheet required to make the funnel (see the given figure).
उत्तर

Radius (r1) of upper circular end of frustum part = 18/2 = 9 cm
Radius (r2) of lower circular end of frustum part = Radius of circular end of cylindrical part
= 8/2 = 4 cm
Height (h1) of frustum part = 22 − 10 = 12 cm
Height (h2) of cylindrical part = 10 cm
Slant height (l) of frustum part =
` sqrt(r_1-r_2^2+h_2) = sqrt((9-4)^2+(12)^2) = 13 cm`
Area of tin sheet required = CSA of frustum part + CSA of cylindrical part
`=pi(r_1+r_2)l+2pir_2h_2`
`=22/7xx(9+4)xx13+22/7xx4xx10`
`=22/7[169+80] = (22xx249)/7`
`=782 4/7 cm^2`
APPEARS IN
संबंधित प्रश्न
The dimensions of a solid iron cuboid are 4·4 m × 2·6 m × 1·0 m. It is melted and recast into a hollow cylindrical pipe of 30 cm inner radius and thickness 5 cm. Find the length of the pipe
Water flows through a cylindrical pipe , whose inner radius is 1 cm , at the rate of 80 cm /sec in an empty cylindrical tank , the radius of whose base is 40 cm . What is the rise of water level in tank in half an hour ?
In the middle of a rectangular field measuring 30 m × 20 m, a well of 7 m diameter and 10 m depth is dug. The earth so removed is evenly spread over the remaining part of the field. Find the height through which the level of the field is raised.
If the heights of two right circular cones are in the ratio 1 : 2 and the perimeters of their bases are in the ratio 3 : 4, what is the ratio of their volumes?
The radii of two cones are in the ratio 2 : 1 and their volumes are equal. What is the ratio of their heights?
A spherical ball of diameter 21 cm is melted and recast into cubes, each of side 1 cm. Find the number of cubes so formed.
The volume of a sphere is 4851 cm3. Find its curved surface area.
A toy is in the form of a cone mounted on a hemisphere of common base radius 7 cm. The total height of the toy is 31 cm. Find the total surface area of the toy.
The surface areas of a sphere and a cube are equal. Find the ratio of their volumes.
Match the column:
| (1) surface area of cuboid | (A) πr2h |
| (2) surface area of closed right cylinder | (B) 2πr(h + r) |
| (3) Total surface area of right cone | (C) πrl + πr2 |
| (4) Total surface area of hemisphere | (D) 3πr3 |
| (E) 3πr2 | |
| (F) 2[lb + bh + lh] |
