Question

As observed from the top of a 75 m high lighthouse from the sea level, the angles of depression of two ships are 30° and 45°. If one ship is exactly behind the other on the same side of the lighthouse, find the distance between the two ships. `("Use"  sqrt3 = 1.732)`

Answer 1

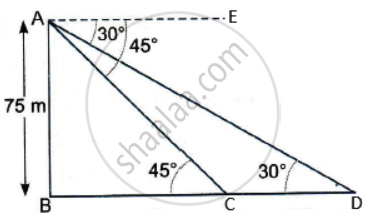
Let AB be the lighthouse and the two ships be at points C and D, respectively.

In ΔABC,

tan 45° = `(AB)/(BC)`

1 = `(75m)/(BC)`

BC = 75 m

In ΔABD,

tan 60° = `(AB)/(BD)`

`1/sqrt3 = 75/(BC + CD)`

`1/sqrt3 = 75/(75 + CD)`

75 + CD = `75sqrt3`

CD = `75 sqrt3 - 75`

CD = `75(sqrt3-1)`

CD = 75(1.732 − 1)

CD = 75 × 0.732

∴ CD = 54.9 m

Hence, the Distance between the two ships is 54.9 m.