Advertisements
Advertisements
प्रश्न
Calculate the centripetal acceleration of the Moon towards the Earth.
Advertisements
उत्तर
The centripetal acceleration is given by a = `v^2/r` This expression explicitly depends on Moon’s speed which is nontrivial. We can work with the formula
ω2Rm = am
am is the centripetal acceleration of the Moon due to Earth’s gravity, ω is the angular velocity
Rm is the distance between Earth and the Moon, which is 60 times the radius of the Earth.
Rm = 60R = 60 x 6.4 x 106 = 384 x 106 m
As we know the angular velocity ω = `(2π)/T` and T =
27.3 days = 27.3 x 24 x 60 x 60 second = 2.358 x 106 sec.
By substituting these values in the formula for acceleration
a6 = `((4π^2)(384×10^6))/(2.358×10^8)^2` = 0.00272 ms-2
APPEARS IN
संबंधित प्रश्न
Force acting on the particle moving with constant speed is ______
Choose the correct statement from the following.
What is the meaning of ‘pseudo force’?
Under what condition will a car skid on a leveled circular road?
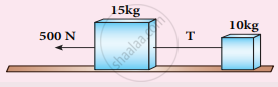
Two bodies of masses 15 kg and 10 kg are connected with light string kept on a smooth surface. A horizontal force F=500 N is applied to a 15 kg as shown in the figure. Calculate the tension acting in the string?
Imagine that the gravitational force between Earth and Moon is provided by an invisible string that exists between the Moon and Earth. What is the tension that exists in this invisible string due to Earth’s centripetal force? (Mass of the Moon = 7.34 × 1022 kg, Distance between Moon and Earth = 3.84 × 108 m)
Explain the need for banking of tracks.
Briefly explain ‘centrifugal force’ with suitable examples.
Explain the similarities and differences of centripetal and centrifugal forces.
Three point masses, each of mass ‘m’ are kept at the corners of an equilateral triangle of side ‘L’. The system rotates about the center of the triangle without any change in the separation of masses during rotation. The period of rotation is directly proportional to `(cos 30° = (sqrt3)/2)` ______.
