Advertisements
Advertisements
प्रश्न
Construct `overline"AB"` of length 7.8 cm. From this, cut off `overline"AC"` of length 4.7 cm. Measure `overline"BC"`.
उत्तर
- Draw a line l and mark a point A on it.

-
By adjusting the compasses up to 7.8 cm, draw an arc to cut l on B, while putting the pointer of compasses on point A.
`overline"AB"` is the line segment of 7.8 cm.

- By adjusting the compasses up to 4.7 cm, draw an arc to cut l on C, while putting the pointer of compasses on point A. `overline"AC"` is the line segment of 4.7 cm.

- Now, put the ruler along with this line such that 0 mark of the ruler will match with point C.
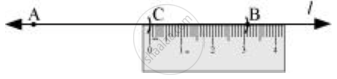
On reading the position of point B, it comes to 3.1 cm. `overline"BC"` is 3.1 cm.
APPEARS IN
संबंधित प्रश्न
Draw any circle and mark points A, B and C such that
- A is on the circle.
-
B is in the interior of the circle.
- C is in the exterior of the circle.
Draw a line segment of length 7.3 cm using a ruler.
Construct a line segment of length 5.6 cm using ruler and compasses.
Given `overline"AB"` of length 3.9 cm, construct `overline"PQ"` such that the length of `overline"PQ"` is twice that of `overline"AB"`. Verify by measurement.

(Hint: construct `overline"PX"` such that length of `overline"PX"` = length of `overline"AB"`; then cut off `overline"XQ"` such that `overline"XQ"` also has the length of `overline"AB"`.)
Given `overline"AB"` of length 7.3 cm and `overline"CD"` of length 3.4 cm, construct a line segment `overline"XY"` such that the length of `overline"XY"` is equal to the difference between the lengths of `overline"AB"` and `overline"CD"`. Verify by measurement.
