Question

Draw a triangle ABC with side BC = 6cm, ∠B = 45° and ∠A = 100°, then construct a triangle PBQ whose sides are `7/4` times the corresponding sides of ΔABC.

Answer 1

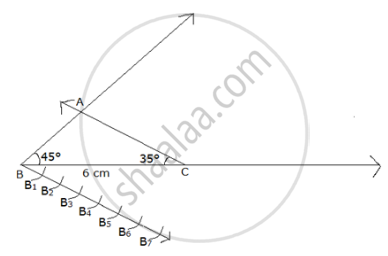
Step-1: construct base BC = 6 cm

Step-2: using protractor draw a line at 45° from point B

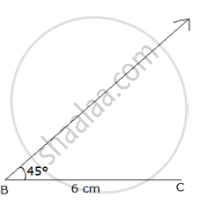
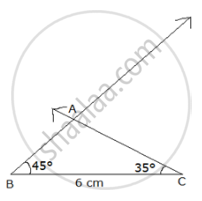
Step-3: given ∠A = 100° since sum of all angles in a triangle is 180° ∴ ∠A + ∠B + ∠C = 180°

∴ 100° + 45° + ∠C = 180°

∴ ∠C = 180° - 145° = 35°

Hence construct a line at 35° from point C

Mark the intersection point as A and ∆ABC is ready

Step-4: construct a line ‘l’ below BC at any angle and also extend the segment BC

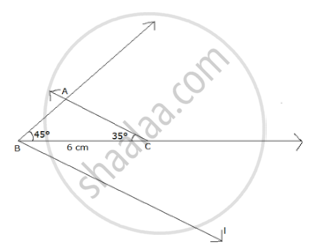
Step-5: take any distance in compass and keep the needle of compass at point B construct an arc and name the point as B₁ then keep the needle at B₁ and draw an arc and name the point as B₂. Repeat the process till B₇. By doing this we are dividing the line into 7 equal parts

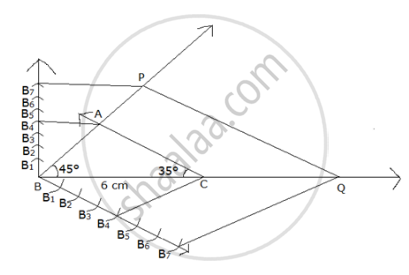
Step-6: join points CB₄ and construct a line parallel to CB₄ from B₇ on the extended line BC mark the intersection point as Q

Step-7: repeat Step5 by constructing a line at any angle to line BA

Step-8: join B₄ and A and construct a line parallel to AB₄ from B₇ on line AB mark the intersection point as P

Join points P and Q required ∆PBQ is ready