Advertisements
Advertisements
प्रश्न
Draw the rough sketch of the curve y = 20 cos2x; `("where" pi/6 ≤ x ≤ pi/3)`.
Using integration, find the area of the region bounded by the curve y = 20 cos2x from the coordinates `x = pi/6` to `x = pi/3` and the x-axis.
आलेख
योग
उत्तर
`y = 20 cos2x; {pi/6 ≤ x ≤ pi/3}`
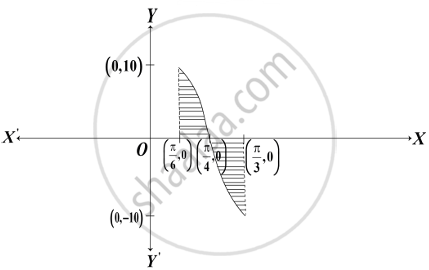
Required area = `20int_(pi/6)^(pi/4) cos2x dx + |20 int_(pi/4)^(pi/3) cos2x dx|`
= `20[(sin2x)/2]_(pi/6)^(pi/4) + |20[(sin2x)/2]_(pi/4)^(pi/3)|`
= `10(1 - sqrt3/2) + 10(1 - sqrt3/2)`
= `20(1 - sqrt3/2)` sq. units
shaalaa.com
क्या इस प्रश्न या उत्तर में कोई त्रुटि है?
