Advertisements
Advertisements
प्रश्न
Explain the origin of magnetism in material, hence find a magnetic moment of an electron revolving around the nucleus of an atom.
उत्तर
- Origin of magnetism in material:
a. Magnetism has its origin in the circulating charges in an atom.
b. Circulating electron is equivalent to a current loop and has a magnetic dipole moment.
c. An atom of any substance consists of a small massive positively charged nucleus surrounded by negatively charged electrons revolving in a circular orbit around the nucleus.
d. The magnetic moment is associated with the motion of an electron in its orbit and is termed an orbital magnetic moment. - Expression for magnetic dipole moment:
a. Consider an electron of mass me and charge e revolving in a circular orbit of radius r around the positive nucleus in the clockwise direction, leading to an anticlockwise current.
U.C.M of an electron around the nucleus
b. If the electron travels a distance 2πr in time T then, its orbital speed v = 2πr/T
c. The magnitude of circulating current is given by,
I = e`(1/"T")`
But, T = `(2pi"r")/"v"`
∴ I = e`(1/(2pi"r""/""v")) = "ev"/(2pi"r")`
d. The orbital magnetic moment associated with the orbital current loop is given by,
morb = IA = `"ev"/(2pi"r") xx pi"r"^2` [∵ Area of current loop, A = πr2]
∴ morb = `"evr"/2` ….(1)
e. The angular momentum of an electron due to its orbital motion is given by, L = mevr
f. Multiplying and dividing the R.H.S of equation (1) by me,
morb = `"e"/(2"m"_"e") xx "m"_"e""vr"`
∴ morb = `"eL"/(2"m"_"e")`
g. This equation shows that orbital magnetic moment is proportional to the angular momentum. But as the electron bears negative charge, the orbital magnetic moment and orbital angular momentum are in opposite directions and perpendicular to the plane of the orbit.
Using vector notation, `vec"m"_"orb" = -("e"/(2"m"_"e"))vec"L"`
APPEARS IN
संबंधित प्रश्न
An electron in an atom is revolving around the nucleus in a circular orbit of a radius of 5.3 x 10-11 m, with a speed of 2 x 106 m/s. Find the resultant orbital magnetic moment and angular momentum of the electron. [e = 1.6 x 10-19 C, me= 9.1 x 10-31 kg]
What is stated in terms of Bohr magneton?
The electron in the hydrogen atom is moving with a speed of 2.5 × 106 m/s in an orbit of a radius of 0.5 Å. What is the Magnetic moment of the revolving electron?
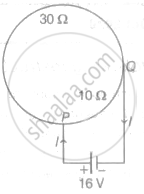
A circular coil of radius R has a resistance of 40 Ω. Figure shows two points P and Q on the circumference separated by a distance `(pi"R")/2`, which are connected to a 16 V battery with internal resistance of 0.5 Ω. What is the value of current I flowing through the circuit?
The magnetic moment of electrons due to orbital motion is proportional to ______.
(n = principal quantum numbers)
The S.I. unit of gyromagnetic ratio is ______
The Gyromagnetic ratio of the electron revolving in a circular orbit of a hydrogen atom is 8.8 x 1010 C kg-1. What is the mass of the electron? (Given charge of the electron = 1.6 x 10-19 C.)
The angle made by orbital angular momentum of electron with the direction of the orbital magnetic moment is ______.
Dimensions of Gyromagnetic ratio are _________.
An electron revolving in a circular orbit of radius 'r' with velocity 'v' and frequency v has orbital magnetic moment 'M'. If the frequency of revolution is tripled then the new magnetic moment will be ____________.
The electron in the hydrogen atom is moving with a speed of 1.5 x 106 m/s in an orbit of radius 2 Å. Magnetic moment of the revolving electron is ____________.
In a hydrogen atom, an electron of charge e revolves in a orbit of radius r with speed v. Then, the magnetic moment associated with electron is ______.
An electron revolving in a circular orbit of radius 'r' with velocity 'V' and frequency 'v' has orbital magnetic moment 'M'. If the frequency of revolution is doubled then the new magnetic moment will be ____________.
An electron (e) is revolving in a circular orbit of radius 'r' in hydrogen atom. The angular momentum of the electron is (M = magnetic dipole moment associated with it and m = mass of electron) ____________.
An electron in the ground state of hydrogen atom is revolving in a circular orbit of radius R. The orbital magnetic moment of the electron is ____________. (m = mass of electron, h = Planck's constant, e = electronic charge)
The electron in hydrogen atom is moving in an orbit of radius 0.53 Å. It takes 1.571 x 10-16 s to complete one revolution. The velocity of electron will be `pi` = 3.142.
In an atom, electron of charge (-e) perform U.C.M. around a stationary positively charged nucleus, with period of revolution 'T'. If 'r' is the radius of the orbit of the electron and 'v' is the orbital velocity, then the circulating current (I) is proportional to ____________.
The period of oscillation of a magnet in a vibration magnetometer is 2 sec. The period of oscillation of a magnet whose magnetic moment is four times that of the first magnet is ______.
The electron in the hydrogen atom is moving with a speed at 2.3 x 106 m/s in an orbit of radius 0.53Å. Calculate the magnetic moment of the revolving electron.
