Advertisements
Advertisements
प्रश्न
Find all the zeroes of polynomial `(2x^4 – 11x^3 + 7x^2 + 13x – 7)`, it being given that two of its zeroes are `(3 + sqrt2) and (3 – sqrt2)`.
उत्तर
The given polynomial is f(x) = `2x^4 – 11x^3 + 7x^2 + 13x – 7.`
Since `(3 + sqrt2) and (3 – sqrt2)` are the zeroes of f(x) it follows that each one of `(x + 3 + sqrt2) and (x + 3 – sqrt2) `is a factor of f(x).
Consequently,` [(x – ( 3 + sqrt2)] [(x – (3 – sqrt2)] = [(x – 3) - sqrt2 ] [(x – 3) + sqrt2 ]`
=`[(x – 3)^2 – 2 ] = x^2 – 6x + 7,` which is a factor of f(x).
On dividing f(x) by `(x^2 – 6x + 7)`, we get:
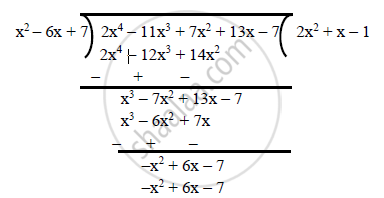
f(x) = 0
⇒` 2x^4 – 11x^3 + 7x^2 + 13x – 7 = 0`
⇒ `(x^2 – 6x + 7) (2x2 + x – 7) = 0`
⇒` (x + 3 + sqrt2) (x + 3 – sqrt2) (2x – 1) (x + 1) = 0`
⇒ `x = –3 – sqrt2 or x = –3 + sqrt2 or x =1/2 or x = -1`
Hence, all the zeroes are `(–3 – sqrt2), (–3 + sqrt2),1/2 and -1.`
APPEARS IN
संबंधित प्रश्न
One zero of the polynomial `3x^3+16x^2 +15x-18 is 2/3` . Find the other zeros of the polynomial.
Find ∝ , β are the zeros of polynomial ∝ +β= 6 and ∝β 4 then write the polynomial.
Write the zeros of the polynomial `f(x) = x^2 – x – 6`.
If 𝛼, 𝛽 are the zeroes of the polynomial `f(x) = x^2 – 5x + k` such that 𝛼 - 𝛽 = 1, find the value of k = ?
A quadratic polynomial, whose zeores are -4 and -5, is ______.
If p(x) is a polynomial of at least degree one and p(k) = 0, then k is known as ______.
Consider the following statements.
- x – 2 is a factor of x3 – 3x² + 4x – 4.
- x + 1 is a factor of 2x3 + 4x + 6.
- x – 1 is a factor of x5 + x4 – x3 + x² -x + 1.
In these statements
The number of polynomials having zeroes as -2 and 5 is ______.
The number of quadratic polynomials having zeroes –5 and –3 is ______.
The number of polynomials having zeroes – 3 and 4 is ______.
