Advertisements
Advertisements
प्रश्न
Find the area of the cardboard required to make a closed box of length 25 cm, 0.5 m and height 15 cm.
उत्तर
\[\text { Length of the box }= 25 cm \]
\[\text { Width of the box = 0 . 5 m }\]
\[ = 0 . 5 \times 100 cm ( \because 1 m = 100 cm)\]
\[ = 50 cm \]
\[\text { Height of the box = 15 cm }\]
\[ \therefore \text { Surface area of the box = 2 }\times\text { (length }\times \text { breadth + breadth }\times \text { height + length } \times \text { height)} \]
\[ = 2 \times (25 \times 50 + 50 \times 15 + 25 \times 15)\]
\[ = 2 \times (1250 + 750 + 375)\]
\[ = 4750 {cm}^2\]
APPEARS IN
संबंधित प्रश्न
A closed iron tank 12 m long, 9 m wide and 4 m deep is to be made. Determine the cost of iron sheet used at the rate of Rs. 5 per metre sheet, sheet being 2 m wide.
The cost of preparing the walls of a room 12 m long at the rate of Rs. 1.35 per square metre is Rs. 340.20 and the cost of matting the floor at 85 paise per square metre is Rs. 91.80. Find the height of the room.
A tank is 8 m long, 6 m broad and 2 m high. How much water can it contain?
If V is the volume of a cuboid of dimensions a, b, c and S is its surface area, then prove that \[\frac{1}{V} = \frac{2}{S}\left( \frac{1}{a} + \frac{1}{b} + \frac{1}{c} \right)\]
The breadth of a room is twice its height, one half of its length and the volume of the room is 512 cu. dm. Find its dimensions.
The dimensions of a Cinema Hall are 100 m, 60 m, and 15 m. How many persons can sit in the hall if each requires 150 m3 of air?
A closed rectangular box is made of wood of 1.5 cm thickness. The exterior length and breadth are respectively 78 cm and 19 cm, and the capacity of the box is 15 cubic decimeters. Calculate the exterior height of the box.
The sum of the radius and the height of a cylinder is 37 cm and the total surface area of the cylinder is 1628 cm2. Find the height and the volume of the cylinder.
The dimensions of a hall is 10 m × 9 m × 8 m. Find the cost of white washing the walls and ceiling at the rate of ₹ 8.50 per m2
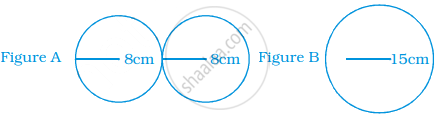
Below are the drawings of cross sections of two different pipes used to fill swimming pools. Figure A is a combination of 2 pipes each having a radius of 8 cm. Figure B is a pipe having a radius of 15 cm. If the force of the flow of water coming out of the pipes is the same in both the cases, which will fill the swimming pool faster?