Advertisements
Advertisements
प्रश्न
Find the smallest number by which the given number must bew multiplied so that the product is a perfect square:
7688
उत्तर
Factorise each number into its prime factors.
7688 = 2 x 2 x 2 x 31 x 31
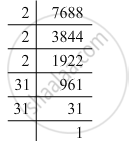
Grouping 7688 into pairs of equal factors:
7688 = (2 x 2) x (31 x 31) x 2
Here, 2 does not occur in pairs. To be a perfect square, every prime factor has to be in pairs. Hence, the smallest number by which 7688 must be multiplied is 2.
APPEARS IN
संबंधित प्रश्न
For the following number, find the smallest whole number by which it should be multiplied so as to get a perfect square number. Also find the square root of the square number so obtained.
1008
For the following number, find the smallest whole number by which it should be divided so as to get a perfect square number. Also find the square root of the square number so obtained.
2645
For the following number, find the smallest whole number by which it should be divided so as to get a perfect square number. Also find the square root of the square number so obtained.
2800
Write true (T) or false (F) for the following statement.
There are fourteen square number upto 200.
Find the square root of each of the following by prime factorization.
441
Find the square root the following by prime factorization.
7056
Find the square root the following by prime factorization.
24336
Find the smallest number by which 147 must be multiplied so that it becomes a perfect square. Also, find the square root of the number so obtained.
Find the smallest number by which 1152 must be divided so that it becomes a perfect square. Also, find the square root of the number so obtained.
Find the least square number, exactly divisible by each one of the number:
8, 12, 15 and 20
