Advertisements
Advertisements
प्रश्न
Find the G.C.D. of the given polynomials
3x3 + 3x2 + 3x + 3, 6x3 + 12x2 + 6x + 12
उत्तर
p(x) = 3x3 + 3x2 + 3x + 3
= 3(x3 + x2 + x + 1)
g(x) = 6x3 + 12x2 + 6x + 12
= 6(x3 + 2x2 + x + 2)
G.C.D. of 3 and 6 = 3
Now g(x) is divided by p(x)
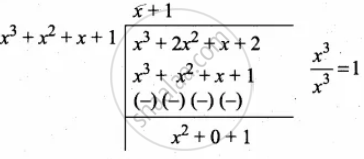
Now dividing p(x) by the remainder x2 + 1
we get x + 1

∴ G.C.D. = 3(x2 + 1) ...[3 is the G.C.D. of 3 and 6]
APPEARS IN
संबंधित प्रश्न
Find the G.C.D. of the given polynomials
x4 + 3x3 – x – 3, x3 + x2 – 5x + 3
Find the G.C.D. of the given polynomials
x4 – 1, x3 – 11x2 + x – 11
Find the L.C.M. of the given expressions
4x2y, 8x3y2
Find the L.C.M. of the given expressions
– 9a3b2, 12a2b2c
Find the L.C.M. of the given expressions
2x2 – 5x – 3, 4x2 – 36
Find the L.C.M. of the given expressions
(2x2 – 3xy)2, (4x – 6y)3, (8x3 – 27y3)
Find the LCM pair of the following polynomials
x4 – 27a3x, (x – 3a)2 whose GCD is (x – 3a)
Find the GCD pair of the following polynomials
12(x4 – x3), 8(x4 – 3x3 + 2x2) whose LCM is 24x3 (x – 1)(x – 2)
Given the LCM and GCD of the two polynomials p(x) and q(x) find the unknown polynomial in the following table
| LCM | GCD | p(x) | q(x) |
| (x4 – y4)(x4 + x2y2 + y2) | (x2 – y2) | (x4 – y4)(x2 + y2 – xy) |
Find the GCD of the following by division algorithm
2x4 + 13x3 + 27x2 + 23x + 7, x3 + 3x2 + 3x + 1, x2 + 2x + 1
