Advertisements
Advertisements
प्रश्न
Find the length of the side of a square if the length of its diagonal is 10 cm.
उत्तर
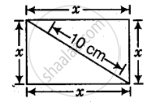
Given, length of diagonal = 10 cm
Suppose, the length of side of a square is x cm.
By using Pythagoras theorem,
(10)2 = x2 + x2
⇒ 100 = 2x2
⇒ x2 = 50
⇒ x = `sqrt(50)` ...[Taking square root on both sides]
∴ x = `5sqrt(2)` cm
Hence, the length of the side of square is `sqrt(50)` or `5sqrt(2)` cm.
APPEARS IN
संबंधित प्रश्न
What will be the units digit of the square of the following number?
52698
From the pattern, we can say that the sum of the first n positive odd numbers is equal to the square of the n-th positive number. Putting that into formula:
1 + 3 + 5 + 7 + ... n = n2, where the left hand side consists of n terms.
Which of the following triplet is pythagorean?
(18, 80, 82)
Observe the following pattern \[1 = \frac{1}{2}\left\{ 1 \times \left( 1 + 1 \right) \right\}\]
\[ 1 + 2 = \frac{1}{2}\left\{ 2 \times \left( 2 + 1 \right) \right\}\]
\[ 1 + 2 + 3 = \frac{1}{2}\left\{ 3 \times \left( 3 + 1 \right) \right\}\]
\[1 + 2 + 3 + 4 = \frac{1}{2}\left\{ 4 \times \left( 4 + 1 \right) \right\}\]
and find the values of following:
1 + 2 + 3 + 4 + 5 + ... + 50
Find the square of the following number:
862
Find the square of the following number:
95
If one member of a pythagorean triplet is 2m, then the other two members are ______.
The sum of first n odd natural numbers is ______.
If x and y are integers such that x2 > y2, then x3 > y3.
Can a right triangle with sides 6 cm, 10 cm and 8 cm be formed? Give reason.
